Unit outcomes

By the end of this unit you will be able to:

• Use the simple growth formula to solve problems related to finance.
• Solve for [latex]\scriptsize A,P,i[/latex] and [latex]\scriptsize n[/latex] using the simple interest formula.

What you should know

Before you start this unit, make sure that you can:

• Use a scientific calculator. Look back to level 2 subject outcome 1.1 to revise this.
• Use basic laws of arithmetic.
• Solve simple equations. Level 2 subject outcome 2.2 unit 1 will provide help with these.
• Complete basic simple interest calculations. Refer to level 2 subject outcome 5.2 unit 1 for help with this.

Introduction

Simple and compound growth formulae are used most commonly when talking about financial loans and investments. Growth is calculated as ‘interest’ on an amount that is invested or borrowed for a period of time. In this unit we will look at simple interest and how it is calculated.

Calculating simple interest

The elements of the simple interest/growth formula are as follows:

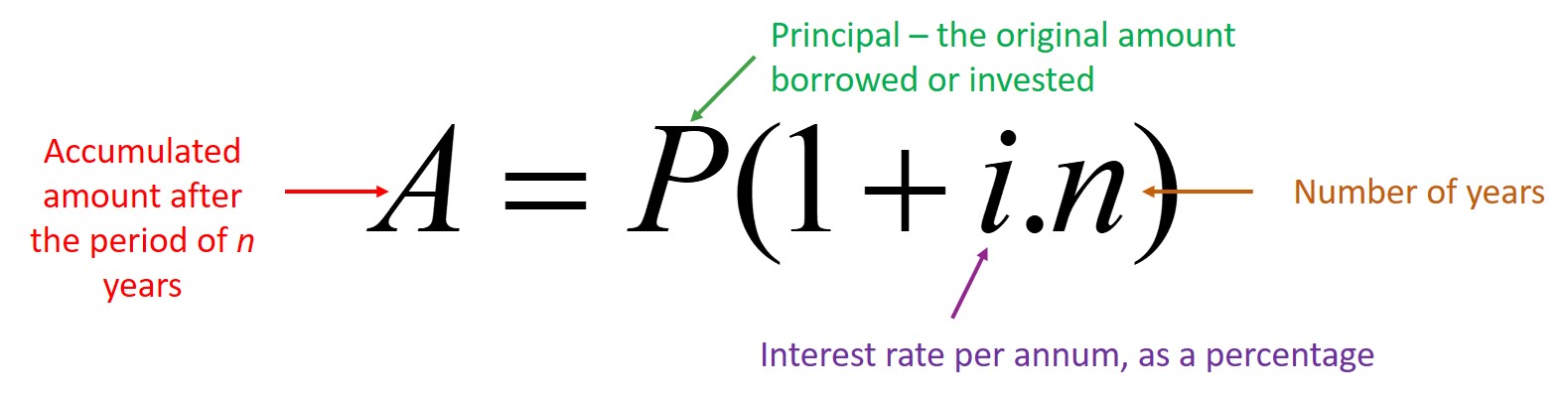
If you multiply out the brackets in this formula, you see that the accumulated amount is made up of the sum of the principal – the original amount that is borrowed or invested – and the increase (by the given percentage) of the principal over the specified number of years:

[latex]\scriptsize A=P+P.i.n[/latex]

Let’s look at a few examples of how to use this simple interest formula.

Example 1.1

A TV costs [latex]\scriptsize \text{R}4\text{ 500}[/latex] on a hire purchase loan agreement. The interest charged is [latex]\scriptsize 12\%[/latex] simple interest each year (per annum, or ‘p.a.’) based on the full purchase price of the TV. Portia repays the loan over three years.

1. Calculate the full amount of money paid for the TV.
2. Calculate how much must be paid each month if equal payments are made every month.

Solution

It is a good idea to list all the information given, and to identify what variable must be calculated.

[latex]\scriptsize \begin{align*}P&=4\text{ }500\\i&=12\%\\n&=3\\A&=?\end{align*}[/latex]

The interest is always a percentage. You can express this as a fraction over [latex]\scriptsize 100[/latex], or substitute the decimal value right away: [latex]\scriptsize 12\%=\displaystyle \frac{{12}}{{100}}=0.12[/latex]

1. .
   [latex]\scriptsize \begin{align*}A&=P(1+i.n)\\&=4\text{ }500\left[ {1+\left( {\displaystyle \frac{{12}}{{100}}} \right)\left( 3 \right)} \right]\\&=4\text{ }500\left[ {1+0.36} \right]\\&=4\text{ }500(1.36)\\&=6\text{ }120\end{align*}[/latex]
   A total of [latex]\scriptsize \text{R}6\text{ 120}[/latex] is paid over the period of three years or [latex]\scriptsize 36[/latex] months.
2. Each monthly payment should be [latex]\scriptsize \displaystyle \frac{{6\text{ }120}}{{36}}=\text{R}170[/latex]

Example 1.2

Pumla borrows [latex]\scriptsize \text{R}150\text{ 000}[/latex] to start a business and repays the loan in one lump sum after five years. The total amount that she is required to pay is [latex]\scriptsize \text{R}243\text{ 750}[/latex]. If the interest charged on the loan was simple interest, calculate the annual interest rate.

Solution

List all the information given and identify what variable must be calculated:
[latex]\scriptsize \begin{align*}P&=150\text{ }000\\A&=243\text{ }750\\n&=5\\i&=?\end{align*}[/latex]

Therefore:

[latex]\scriptsize \begin{align*}A&=P(1+i.n)\\ \displaystyle \frac{A}{P}&=1+i.n\\ \displaystyle \frac{A}{P}-1&=i.n\\ \displaystyle \frac{1}{n}\left( {\displaystyle \frac{A}{P}-1} \right)&=i\\ i&=\displaystyle \frac{1}{5}\left( {\displaystyle \frac{{243\text{ }750}}{{150\text{ }000}}-1} \right)\\ &=\displaystyle \frac{1}{5}\left( {1.625-1} \right)\\ &=\displaystyle \frac{1}{5}\left( {0.625} \right)\\ &=0.125\text{ or 12}\text{.5 }\end{align*}[/latex]

Example 1.3

A cell phone is advertised for [latex]\scriptsize \text{R}280[/latex] per month on hire purchase over a period of three years. If the simple interest rate charged is [latex]\scriptsize 33.9\%[/latex], calculate the original cash price of the phone. What would the buyer save by buying the phone for cash rather than on hire purchase?

Solution

List all the information given and identify what variable must be calculated:

[latex]\scriptsize \begin{align*}n&=3\text{ years}\\i&=33.9\%\\A&=280\times (3\times 12)=10\ 080\\P&=?\end{align*}[/latex]

Therefore:

[latex]\scriptsize \begin{align*}A&=P(1+i.n)\\\displaystyle \frac{A}{{1+i.n}}&=P\\P&=\displaystyle \frac{{10\ 080}}{{1+\left( {\displaystyle \frac{{33.9}}{{100}}} \right)3}}\\&=\displaystyle \frac{{10\ 080}}{{1+(0.339\times 3)}}\\&=\displaystyle \frac{{10\ 080}}{{1+1.017}}\\&=\displaystyle \frac{{10\ 080}}{{2.017}}\\&=4\ 997.52\end{align*}[/latex]

The cash price for the phone is [latex]\scriptsize \text{R}4\text{ 997}\text{.52}[/latex].

The buyer would save [latex]\scriptsize 10\ 080-4\ 997.52=\text{R}5\ 082.48[/latex] by paying cash rather than buying on hire purchase.

Example 1.4

How long will it take for an investment of [latex]\scriptsize \text{R}7\text{ 500}[/latex] to amount to [latex]\scriptsize \text{R}10\text{ 125}[/latex] at [latex]\scriptsize 3.5\%[/latex] simple interest per annum?

Solution

List all the information given and identify what variable must be calculated:

[latex]\scriptsize \begin{align*}P&=7\text{ }500\\A&=10\text{ }125\\i&=3.5\%=\displaystyle \frac{{3.5}}{{100}}=0.035\\n&=?\end{align*}[/latex]

Therefore:

[latex]\scriptsize \begin{align*}A&=P(1+i.n)\\\displaystyle \frac{A}{P}&=1+i.n\\\displaystyle \frac{A}{P}-1&=i.n\\\displaystyle \frac{1}{i}\left( {\displaystyle \frac{A}{P}-1} \right)&=n\\n&=\displaystyle \frac{1}{{0.035}}\left( {\displaystyle \frac{{10\text{ }125}}{{7\text{ }500}}-1} \right)\\&=\displaystyle \frac{1}{{0.035}}(1.35-1)\\&=\displaystyle \frac{1}{{0.035}}(0.35)\\&=10\end{align*}[/latex]
It will take [latex]\scriptsize 10[/latex] years.

Exercise 1.1

Note: Save your calculations and answers from this exercise to compare with your work in unit 2 in this subject outcome.

1. [latex]\scriptsize \text{R}3\text{ }750[/latex] is invested in a savings account at [latex]\scriptsize 3.2\%[/latex] simple interest per annum. What is the total value of the investment after a period of four years?
2. A loan of [latex]\scriptsize \text{R}30\text{ }000[/latex] is offered, to be paid off over a period of two years. By the time the debt is paid off, Karabo has paid a total of [latex]\scriptsize \text{R}9\text{ }000[/latex] in interest. What is the rate of simple interest that was charged on the loan?
3. A laptop is advertised for [latex]\scriptsize \text{R}299[/latex] per month on hire purchase over a period of three years. If the simple interest rate charged is [latex]\scriptsize 35\%[/latex], calculate the original cash price of the laptop. How much would the buyer save by buying the laptop for cash rather than on hire purchase?
4. Calculate how long it would take to generate an amount of [latex]\scriptsize \text{R}1\text{ }050[/latex] in interest from an investment of [latex]\scriptsize \text{R}5\text{ }000[/latex], at [latex]\scriptsize 3.5\%[/latex]simple interest per annum.

The full solutions are at the end of the unit.

Summary

In this unit you have learnt the following:

• How to use the simple growth formula to solve problems related to finance.
• How to solve for [latex]\scriptsize A\text{, }P\text{, }i[/latex] and [latex]\scriptsize n[/latex] using the simple interest formula.

Unit 1: Assessment

Suggested time to complete: 20 minutes

1. A laptop is advertised for [latex]\scriptsize \text{R}469[/latex] per month over a period of three years on hire purchase. If the rate of simple interest charged is [latex]\scriptsize 22.95\%[/latex], calculate the original cash price of the laptop. Calculate the amount saved by buying for cash rather than on hire purchase.
2. After a period of [latex]\scriptsize 20[/latex] years, an investment of [latex]\scriptsize \text{R}12\text{ 750}[/latex] has a total value of [latex]\scriptsize \text{R}23\text{ 205}[/latex]. Calculate the rate of simple interest that was paid on this investment.
3. A new fridge is advertised for [latex]\scriptsize \text{R}262[/latex] per month over a period of two years. If the simple interest charged is [latex]\scriptsize 45.3\%[/latex] per annum, calculate the cash price of the fridge.
4. Calculate the time it will take [latex]\scriptsize \text{R}8\text{ 000}[/latex] to amount to [latex]\scriptsize \text{R}8\text{ 960}[/latex] at [latex]\scriptsize 4\%[/latex] simple interest per annum.

The full solutions are at the end of the unit.

Unit 1: Solutions

Exercise 1.1

1. .
   [latex]\scriptsize P=\text{R}3\text{ 750}[/latex]
   [latex]\scriptsize i=3.2\%[/latex]
   [latex]\scriptsize n=4[/latex]
   [latex]\scriptsize A=?[/latex]
   .
   [latex]\scriptsize \begin{align*}A&=P(1+i.n)\\&=3750\left[ {1+\displaystyle \frac{{3.2}}{{100}}(4)} \right]\\&=3750[1+(0.032)(4)]\\&=3750[1+0.128]\\&=3750[1.128]\\&=4230\end{align*}[/latex]
   The total value of the investment after the period of four years is [latex]\scriptsize \text{R}4\text{ 230}[/latex].
2. .
   [latex]\scriptsize P=30\text{ }000[/latex]
   [latex]\scriptsize n=2[/latex]
   [latex]\scriptsize A=30\text{ }000+9\text{ 000=39 }000[/latex]
   [latex]\scriptsize i=?[/latex]
   .
   [latex]\scriptsize \begin{align*}A&=P(1+i.n)\\ \displaystyle \frac{A}{P}&=1+i.n\\ \displaystyle \frac{A}{P}-1&=i.n\\ \displaystyle \frac{1}{n}\left( {\displaystyle \frac{A}{P}-1} \right)&=i\\ i&=\displaystyle \frac{1}{2}\left( {\displaystyle \frac{{39000}}{{30000}}-1} \right)\\ &=\displaystyle \frac{1}{2}(1.3-1)\\ &=\displaystyle \frac{1}{2}(0.3)\\ &=0.15\text{ or } 15\% \text{ } \end{align*}[/latex]
   The rate of simple interest charged was [latex]\scriptsize 15\%[/latex] .
3. .
   [latex]\scriptsize \begin{align*}n&=3\text{ years = 36 months}\\i&=35\%\\A&=299\times 36=10764\end{align*}[/latex]
   .
   [latex]\scriptsize \begin{align*}A&=P(1+i.n)\\\displaystyle \frac{A}{{(1+i.n)}}&=P\\P&=\displaystyle \frac{{10\text{ }764}}{{1+\left( {\displaystyle \frac{{35}}{{100}}} \right)(3)}}\\&=\displaystyle \frac{{10\text{ }764}}{{1+(0.35)(3)}}\\&=\displaystyle \frac{{10\text{ }764}}{{1+1.05}}\\&=\displaystyle \frac{{10\text{ }764}}{{2.05}}\\&=5\text{ }250.732\end{align*}[/latex]
   The original cash price of the laptop is [latex]\scriptsize \text{R}5\text{ 250}\text{.73}[/latex].
   Buying for cash rather than hire purchase would save [latex]\scriptsize 10\text{ }764-5\text{ }250.73=\text{R}5\text{ }513.27[/latex].
4. .
   [latex]\scriptsize \begin{align*}P&=5\text{ }000\\A&=1\text{ }050+5\text{ }000=6\text{ }050\\I&=3.5\%=\displaystyle \frac{{3.5}}{{100}}=0.035\end{align*}[/latex]
   .
   [latex]\scriptsize \begin{align*}A&=P(1+i.n)\\\displaystyle \frac{A}{P}&=1+i.n\\\displaystyle \frac{A}{P}-1&=i.n\\\displaystyle \frac{1}{i}\left( {\displaystyle \frac{A}{P}-1} \right)&=n\\n&=\displaystyle \frac{1}{{0.035}}\left( {\displaystyle \frac{{6\text{ }050}}{{5\text{ }000}}-1} \right)\\&=\displaystyle \frac{1}{{0.035}}(1.21-1)\\7&=\displaystyle \frac{1}{{0.035}}(0.21)\\&=6\end{align*}[/latex]
   It would take six years.

Back to Exercise 1.1

Unit 1: Assessment

1. .
   [latex]\scriptsize \begin{align*}n&=3\text{ years}=36\text{ months}\\A&=469\times \text{36=16 884}\\i&=22.95\%\\P&=?\end{align*}[/latex]
   .
   [latex]\scriptsize \begin{align*}A&=P(1+i.n)\\\displaystyle \frac{A}{{1+i.n}}&=P\\P&=\displaystyle \frac{{16\text{ }884}}{{1+\left( {\displaystyle \frac{{22.95}}{{100}}} \right)(3)}}\\&=\displaystyle \frac{{16\text{ }884}}{{1+(0.2295)(3)}}\\&=\displaystyle \frac{{16\text{ }884}}{{1+0.6885}}\\&=\displaystyle \frac{{16\text{ }884}}{{1.6885}}\\&=9\text{ }999.408\end{align*}[/latex]
   The cash price of the laptop is [latex]\scriptsize \text{R}9\ 999.41[/latex].
   The amount saved by buying for cash rather than on hire purchase would be [latex]\scriptsize 16884-9999.41=R6884.59[/latex]
2. .
   [latex]\scriptsize \begin{align*}A&=23\text{ }205\\P&=12\text{ }750\\n&=20\\i&=?\end{align*}[/latex]
   .
   [latex]\scriptsize \begin{align*}A&=P(1+i.n)\\\displaystyle \frac{A}{P}&=1+i.n\\\displaystyle \frac{A}{P}-1&=i.n\\\displaystyle \frac{1}{n}\left( {\displaystyle \frac{A}{P}-1} \right)&=i\\i&=\displaystyle \frac{1}{{20}}\left( {\displaystyle \frac{{23\text{ }205}}{{12\text{ }750}}-1} \right)\\&=\displaystyle \frac{1}{{20}}(1.82-1)\\&=\displaystyle \frac{1}{{20}}(0.82)\\&=0.041\text{ or 4}\text{.1 } \% \text{ p}\text{.a}\text{.}\end{align*}[/latex]
   The rate of simple interest paid was [latex]\scriptsize 4.1\%[/latex] per annum.
3. .
   [latex]\scriptsize \begin{align*}i&=45.3\%\\n&=2\text{ years = 24 months}\\A&=262\times 24=6\ 288\end{align*}[/latex]
   .
   [latex]\scriptsize \begin{align*}A&=P(1+i.n)\\\displaystyle \frac{A}{{(1+i.n)}}&=P\\P&=\displaystyle \frac{{6\text{ }288}}{{1+\left( {\displaystyle \frac{{45.3}}{{100}}} \right)(2)}}\\&=\displaystyle \frac{{6\text{ }288}}{{1+(0.453)(2)}}\\&=\displaystyle \frac{{6\text{ }288}}{{1+0.906}}\\&=\displaystyle \frac{{6\text{ }288}}{{1.906}}\\&=3\text{ }299.056\end{align*}[/latex]
   The cash price of the fridge is [latex]\scriptsize \text{R}3\text{ }299.06[/latex]
4. .
   [latex]\scriptsize \begin{align*}P&=8\text{ }000\\A&=8\text{ }960\\i&=4\%=\displaystyle \frac{4}{{100}}=0.04\end{align*}[/latex]
   .
   [latex]\scriptsize \begin{align*}A&=P(1+i.n)\\\displaystyle \frac{A}{P}&=1+i.n\\\displaystyle \frac{A}{P}-1&=i.n\\\displaystyle \frac{1}{i}\left( {\displaystyle \frac{A}{P}-1} \right)&=n\\n&=\displaystyle \frac{1}{{0.04}}\left( {\displaystyle \frac{{8\text{ }960}}{{8\text{ }000}}-1} \right)\\&=\displaystyle \frac{1}{{0.04}}(1.12-1)\\&=\displaystyle \frac{1}{{0.04}}(0.12)\\&=3\end{align*}[/latex]
   It will take three years.

Back to Unit 1: Assessment