Functions and algebra: Use a variety of techniques to sketch and interpret information from graphs of functions
Unit 8: Horizontal transformation of the sine graph
Dylan Busa
Unit outcomes
By the end of this unit you will be able to:
- Sketch functions of the form [latex]\scriptsize y=\sin (\theta +p)[/latex].
- Determine the effects of positive and negative values of [latex]\scriptsize p[/latex] on the sine graph [latex]\scriptsize y=\sin (\theta +p)[/latex].
- Find the value of [latex]\scriptsize p[/latex] from a given sine graph of the form [latex]\scriptsize y=\sin (\theta +p)[/latex].
Remember that the domain of trigonometric functions can be represented as [latex]\scriptsize x[/latex] or [latex]\scriptsize \theta[/latex]. Therefore, [latex]\scriptsize y=\sin x[/latex] and [latex]\scriptsize y=\sin \theta[/latex] are the same function.
What you should know
Before you start this unit, make sure you can:
- Sketch sine functions of the form [latex]\scriptsize y=a\sin \theta +q[/latex]. Refer to level 2 subject outcome 2.1 Unit 5 if you need help with this.
Introduction
So far in this subject outcome, we have been investigating the effects of the values of [latex]\scriptsize a[/latex], [latex]\scriptsize p[/latex] and [latex]\scriptsize q[/latex] on various functions. For example, for the functions [latex]\scriptsize y=a{{(x+p)}^{2}}+q[/latex], [latex]\scriptsize y=\displaystyle \frac{a}{{(x+p)}}+q[/latex] and [latex]\scriptsize y=a.{{b}^{{x+p}}}+q[/latex] we have discovered that [latex]\scriptsize q[/latex] is responsible for a vertical shift of the graph of the function while [latex]\scriptsize p[/latex] is responsible for a horizontal shift such that:
- if [latex]\scriptsize q \gt 0[/latex], the graph shifts [latex]\scriptsize q[/latex] units up
- if [latex]\scriptsize q \lt 0[/latex], the graph shifts [latex]\scriptsize q[/latex] units down
- if [latex]\scriptsize p \gt 0[/latex], the graph shifts [latex]\scriptsize q[/latex] units to the left
- if [latex]\scriptsize p \lt 0[/latex], the graph shifts [latex]\scriptsize q[/latex] units to the right.
Why [latex]\scriptsize q[/latex] results in a vertical shift
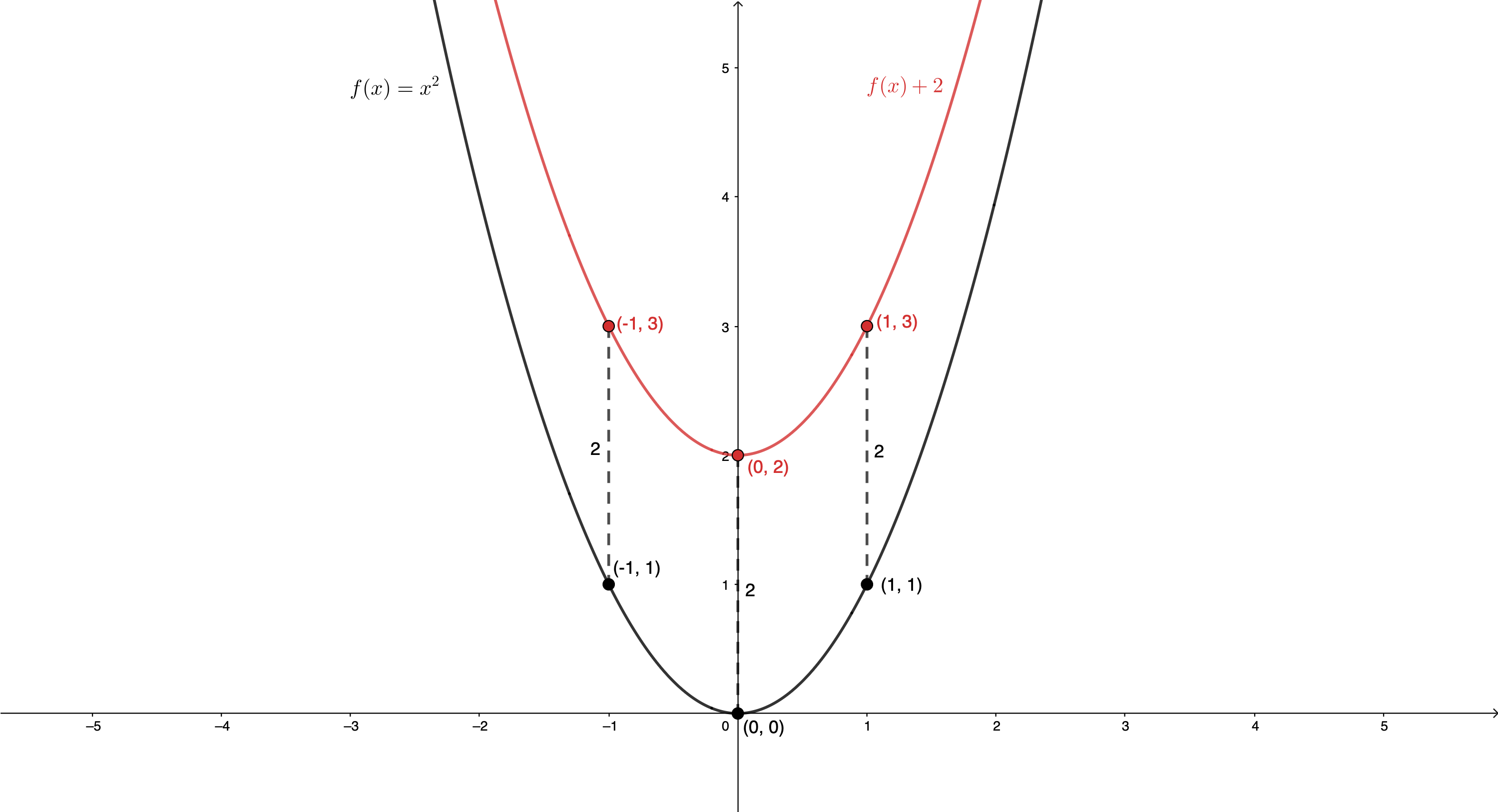
If we consider the basic quadratic function, we can see why this is the case. Consider [latex]\scriptsize f(x)={{x}^{2}}[/latex]. If we add a constant of [latex]\scriptsize q[/latex] to the equation such that it becomes [latex]\scriptsize f(x)={{x}^{2}}+q[/latex], we can see that we will be adding this constant to the value of [latex]\scriptsize f(x)[/latex] (the y-value) for every value of [latex]\scriptsize x[/latex] we feed into the function. In other words, if [latex]\scriptsize f(x)={{x}^{2}}[/latex], then [latex]\scriptsize {{x}^{2}}+q=f(x)+q[/latex]. If [latex]\scriptsize q=2[/latex], for example, we will add this same positive constant to each function and shift the whole graph [latex]\scriptsize 2[/latex] units up (see Figure 1).
Why [latex]\scriptsize p[/latex] results in a horizontal shift
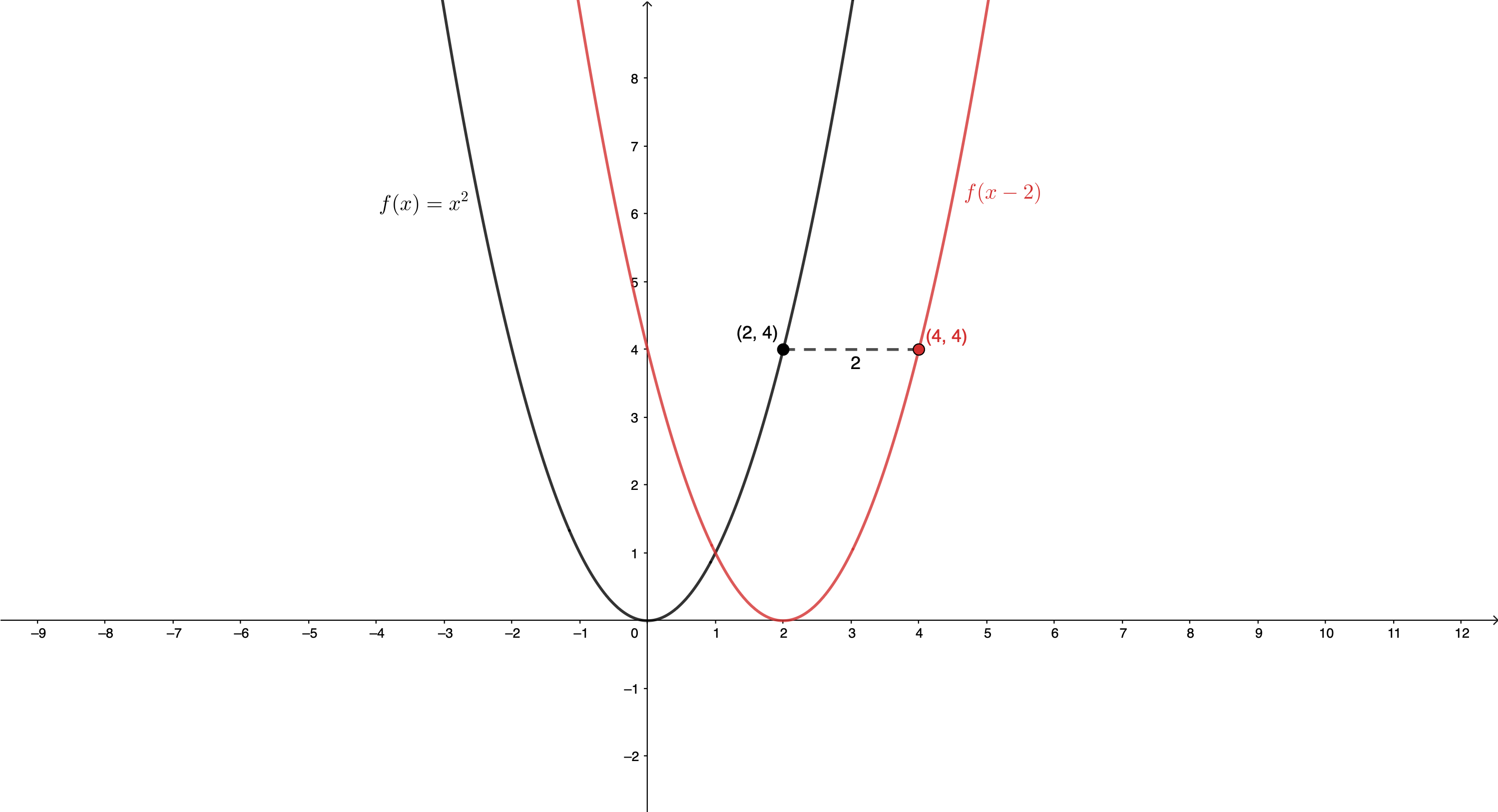
Now let’s consider the role of [latex]\scriptsize p[/latex]. Again, let [latex]\scriptsize f(x)={{x}^{2}}[/latex]. When [latex]\scriptsize x=2[/latex] the function value is [latex]\scriptsize f(2)=2^2=4[/latex]. When [latex]\scriptsize x=4[/latex], the function value is [latex]\scriptsize f(4)=4^2=16[/latex]. But if we replaced [latex]\scriptsize x[/latex] with [latex]\scriptsize (x-2)[/latex] in the function, then the function value when [latex]\scriptsize x=4[/latex] would be [latex]\scriptsize f(4-2)=f(2)=2^2=4[/latex]. In other words, the function value that used to occur when [latex]\scriptsize x=2[/latex] now occurs when [latex]\scriptsize x=4[/latex]. The graph has been shifted two units to the right.
Therefore, replacing [latex]\scriptsize x[/latex] with [latex]\scriptsize (x+p)[/latex] shifts the graph horizontally by [latex]\scriptsize -p[/latex] units. If [latex]\scriptsize p\lt0[/latex], the graph shifts [latex]\scriptsize p[/latex] units to the right. If [latex]\scriptsize p\gt0[/latex], the graph shifts [latex]\scriptsize p[/latex] units to the left.
The sine function of the form [latex]\scriptsize y=\sin (x+p)[/latex]
By looking at the effects of [latex]\scriptsize p[/latex] on the quadratic function above, you should be able to predict the effect that [latex]\scriptsize p[/latex] will have on the graph of [latex]\scriptsize y=\sin (x+p)[/latex]. But let’s investigate.
Activity 8.1: Shifts?
Time required: 40 minutes
What you need:
- a pen or pencil
- a calculator
- several pieces of paper
What to do:
- Start by making a sketch of [latex]\scriptsize y=\sin x[/latex] for the interval [latex]\scriptsize {{0}^\circ}\le x\le {{360}^\circ}[/latex] on your piece of paper. If you need to, you can complete the following table of values first.
[latex]\scriptsize x[/latex] [latex]\scriptsize {{0}^\circ}[/latex] [latex]\scriptsize {{90}^\circ}[/latex] [latex]\scriptsize {{180}^\circ}[/latex] [latex]\scriptsize {{270}^\circ}[/latex] [latex]\scriptsize {{360}^\circ}[/latex] [latex]\scriptsize \sin x[/latex] - Now complete the following tables of values and sketch the additional functions on the same set of axes for the interval [latex]\scriptsize {{0}^\circ}\le x\le {{360}^\circ}[/latex].
[latex]\scriptsize x[/latex] [latex]\scriptsize {{0}^\circ}[/latex] [latex]\scriptsize {{90}^\circ}[/latex] [latex]\scriptsize {{180}^\circ}[/latex] [latex]\scriptsize {{270}^\circ}[/latex] [latex]\scriptsize {{360}^\circ}[/latex] [latex]\scriptsize \sin \left( {x+{{{30}}^\circ}} \right)[/latex] [latex]\scriptsize x[/latex] [latex]\scriptsize {{0}^\circ}[/latex] [latex]\scriptsize {{90}^\circ}[/latex] [latex]\scriptsize {{180}^\circ}[/latex] [latex]\scriptsize {{270}^\circ}[/latex] [latex]\scriptsize {{360}^\circ}[/latex] [latex]\scriptsize \sin \left( {x-{{{60}}^\circ}} \right)[/latex] - Using your sketches, describe the effect of [latex]\scriptsize p[/latex] on the graph of the function [latex]\scriptsize y=\sin (x+p)[/latex].
What did you find?
- Below is the completed table of values and the sketch of [latex]\scriptsize y=\sin x,\text{ }{{0}^\circ}\le x\le {{360}^\circ}[/latex] (see Figure 3).
[latex]\scriptsize x[/latex] [latex]\scriptsize {{0}^\circ}[/latex] [latex]\scriptsize {{90}^\circ}[/latex] [latex]\scriptsize {{180}^\circ}[/latex] [latex]\scriptsize {{270}^\circ}[/latex] [latex]\scriptsize {{360}^\circ}[/latex] [latex]\scriptsize \sin x[/latex] [latex]\scriptsize 0[/latex] [latex]\scriptsize 1[/latex] [latex]\scriptsize 0[/latex] [latex]\scriptsize -1[/latex] [latex]\scriptsize 0[/latex] 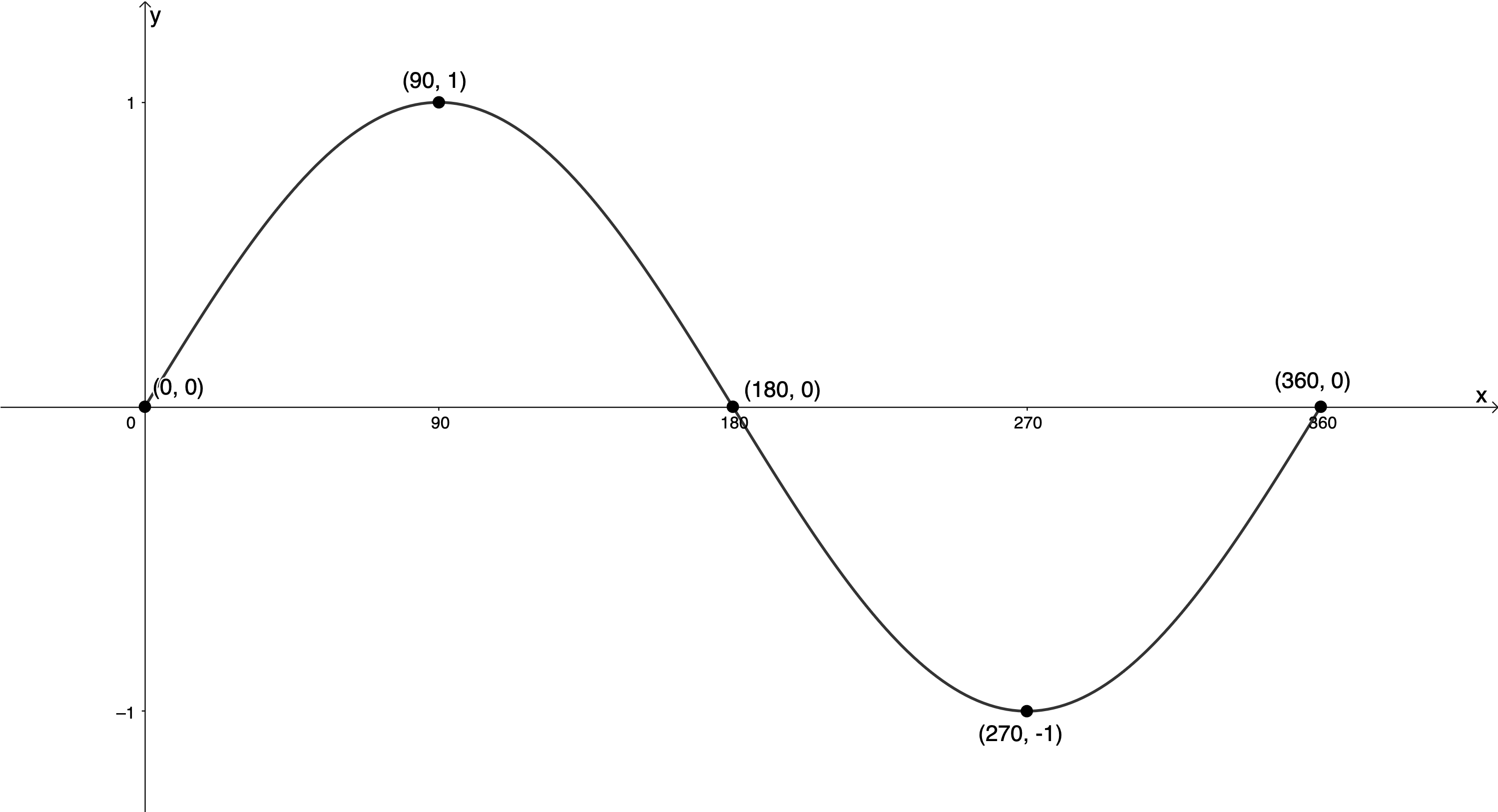
Figure 3: Graph of [latex]\scriptsize y=\sin x,\text{ }{{0}^\circ}\le x\le {{360}^\circ}[/latex] - Here are the completed tables of values for the other functions.
[latex]\scriptsize x[/latex] [latex]\scriptsize {{0}^\circ}[/latex] [latex]\scriptsize {{90}^\circ}[/latex] [latex]\scriptsize {{180}^\circ}[/latex] [latex]\scriptsize {{270}^\circ}[/latex] [latex]\scriptsize {{360}^\circ}[/latex] [latex]\scriptsize \sin \left( {x+{{{30}}^\circ}} \right)[/latex] [latex]\scriptsize \displaystyle \frac{1}{2}[/latex] [latex]\scriptsize 0.866[/latex] [latex]\scriptsize -\displaystyle \frac{1}{2}[/latex] [latex]\scriptsize -0.866[/latex] [latex]\scriptsize \displaystyle \frac{1}{2}[/latex] [latex]\scriptsize x[/latex] [latex]\scriptsize {{0}^\circ}[/latex] [latex]\scriptsize {{90}^\circ}[/latex] [latex]\scriptsize {{180}^\circ}[/latex] [latex]\scriptsize {{270}^\circ}[/latex] [latex]\scriptsize {{360}^\circ}[/latex] [latex]\scriptsize \sin \left( {x-{{{60}}^\circ}} \right)[/latex] [latex]\scriptsize -0.866[/latex] [latex]\scriptsize \displaystyle \frac{1}{2}[/latex] [latex]\scriptsize 0.866[/latex] [latex]\scriptsize -\displaystyle \frac{1}{2}[/latex] [latex]\scriptsize -0.866[/latex] Below are the graphs of [latex]\scriptsize y=\sin x[/latex], [latex]\scriptsize y=\sin \left( {x+{{{30}}^\circ}} \right)[/latex] and [latex]\scriptsize y=\sin \left( {x-{{{60}}^\circ}} \right)[/latex] (see Figure 4).

Figure 4: Graphs of [latex]\scriptsize y=\sin x,\text{ }y=\sin (x+{{30}^\circ}),\text{ }y=\sin (x-{{60}^\circ}),\text{ }{{0}^\circ}\le x\le {{360}^\circ}[/latex] - We can see from the sketches that the value of [latex]\scriptsize p[/latex] in [latex]\scriptsize y=\sin (x+p)[/latex] results in a horizontal shift of the graph left or right by [latex]\scriptsize p[/latex] units. In the case of [latex]\scriptsize y=\sin (x+{{30}^\circ})[/latex] the graph is shifted [latex]\scriptsize {{30}^\circ}[/latex] to the left. In the case of [latex]\scriptsize y=\sin (x-{{60}^\circ})[/latex], the graph is shifted [latex]\scriptsize {{60}^\circ}[/latex] to the right. We can see this by looking at the maximum and minimum turning points of the graphs as well as the x-intercepts
Take note!
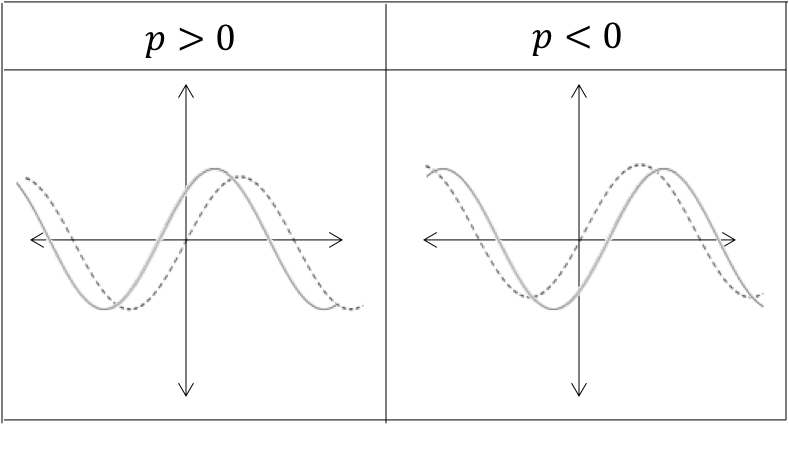
In [latex]\scriptsize y=\sin (x+p)[/latex]:
- if [latex]\scriptsize p \gt 0[/latex], the graph is shifted [latex]\scriptsize p[/latex] units to the left
- if [latex]\scriptsize p \lt 0[/latex], the graph is shifted [latex]\scriptsize p[/latex] units to the right.
Note
If you have an internet connection, spend some time playing with this interactive simulation.
Here you will find a graph of the function [latex]\scriptsize \displaystyle y=\sin (x+p)[/latex] with a slider to change the value of [latex]\scriptsize p[/latex]. Pay particular attention to how changing the value of [latex]\scriptsize p[/latex] affects the location of the turning points and the intercepts with the x-axis.
Sketch functions of the form [latex]\scriptsize y=\sin (x+p)[/latex]
The best way to sketch functions of the form [latex]\scriptsize y=\sin (x+p)[/latex] is to transform the basic function of [latex]\scriptsize y=\sin x[/latex] depending on the value of [latex]\scriptsize p[/latex]. To do this, you need to know the set of ‘anchor points’ of [latex]\scriptsize y=\sin x[/latex], as transformation of these points will help you to sketch functions of the form[latex]\scriptsize y=\sin (x+p)[/latex].
Example 8.1
Given the function [latex]\scriptsize y=\sin (x-{{45}^\circ})[/latex]:
- Sketch the function for the interval [latex]\scriptsize {{0}^\circ}\le x\le {{360}^\circ}[/latex].
- State the domain and range.
- State the period.
- State the amplitude.
Solution
- The function is of the form [latex]\scriptsize y=\sin (x+p)[/latex] with, [latex]\scriptsize p=-{{45}^\circ}[/latex].
We need to transform one period of ‘anchor points’ noting that each of these points is going to be shifted [latex]\scriptsize {{45}^\circ}[/latex] to the right.
.[latex]\scriptsize \sin x[/latex] [latex]\scriptsize ({{0}^\circ},0)[/latex] [latex]\scriptsize ({{90}^\circ},1)[/latex] [latex]\scriptsize ({{180}^\circ},0)[/latex] [latex]\scriptsize ({{270}^\circ},-1)[/latex] [latex]\scriptsize ({{360}^\circ},0)[/latex] [latex]\scriptsize \sin (x-{{45}^\circ})[/latex] [latex]\scriptsize ({{45}^\circ},0)[/latex] [latex]\scriptsize ({{135}^\circ},1)[/latex] [latex]\scriptsize ({{225}^\circ},0)[/latex] [latex]\scriptsize ({{315}^\circ},-1)[/latex] [latex]\scriptsize ({{405}^\circ},0)[/latex] .
To make sure that the shape of the graph is as accurate as possible, we must also find the y-intercept.y-intercept (let [latex]\scriptsize x=0[/latex]):
[latex]\scriptsize \begin{align*}y & =\sin ({{0}^\circ}-{{45}^\circ})\\\therefore y & =-0.707\end{align*}[/latex]
.
Because the period of the function is [latex]\scriptsize {{360}^\circ}[/latex] we know that the function value at [latex]\scriptsize {{360}^\circ}[/latex] will also be [latex]\scriptsize -0.707[/latex].
.
We can now plot our transformed ‘anchor points’ and draw the graph for the interval [latex]\scriptsize {{0}^\circ}\le x\le {{360}^\circ}[/latex].
.
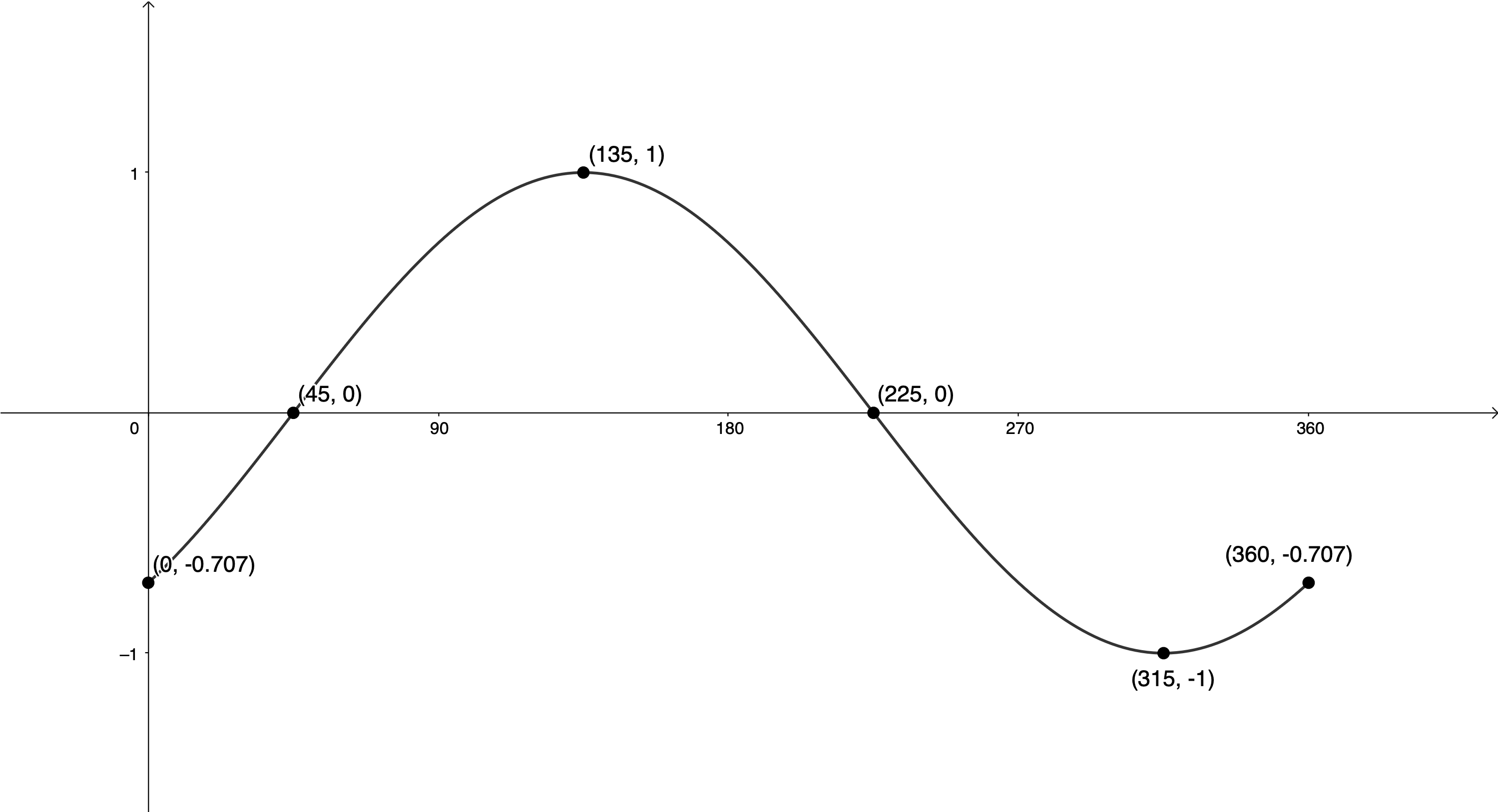
- Domain: [latex]\scriptsize \{x|x\in \mathbb{R},{{0}^\circ}\le x\le {{360}^\circ}\}\text{ }[/latex]
Range: [latex]\scriptsize \{y|y\in \mathbb{R},-1\le x\le 1\}\text{ }[/latex] - The period is [latex]\scriptsize {{360}^\circ}[/latex].
- The amplitude is [latex]\scriptsize 1[/latex].
Take note!
In general, the domain of the function [latex]\scriptsize y=\sin (x+p)[/latex] is [latex]\scriptsize x\in \mathbb{R}\text{ }[/latex]. We had to restrict the domain in Example 8.1, because the interval in which we were working was restricted.
Exercise 8.1
Sketch the following functions for the indicated intervals on separate sets of axes:
- [latex]\scriptsize f(x)=\sin (x+{{30}^\circ})[/latex] for [latex]\scriptsize {{0}^\circ}\le x\le {{360}^\circ}[/latex]
- [latex]\scriptsize g(x)=\sin (x-{{45}^\circ})[/latex] for [latex]\scriptsize -{{360}^\circ}\le x\le {{360}^\circ}[/latex]
- [latex]\scriptsize h(x)=\sin (x+{{20}^\circ})[/latex] for [latex]\scriptsize -{{360}^\circ}\le x\le {{360}^\circ}[/latex]
The full solutions are at the end of the unit.
Sketch functions of the form [latex]\scriptsize y=a\sin (x+p)[/latex]
Now that we know that the value of [latex]\scriptsize p[/latex] shifts the function of the form [latex]\scriptsize y=\sin (x+p)[/latex] horizontally by [latex]\scriptsize p[/latex] degrees, we can combine our knowledge of the effects of [latex]\scriptsize a[/latex] with this and examine functions of the form [latex]\scriptsize y=a\sin (x+p)[/latex].
Example 8.2
Sketch the graph of [latex]\scriptsize f(x)=2\sin (x+{{25}^\circ})[/latex] for the interval [latex]\scriptsize -{{360}^\circ}\le x\le {{360}^\circ}[/latex].
Solution
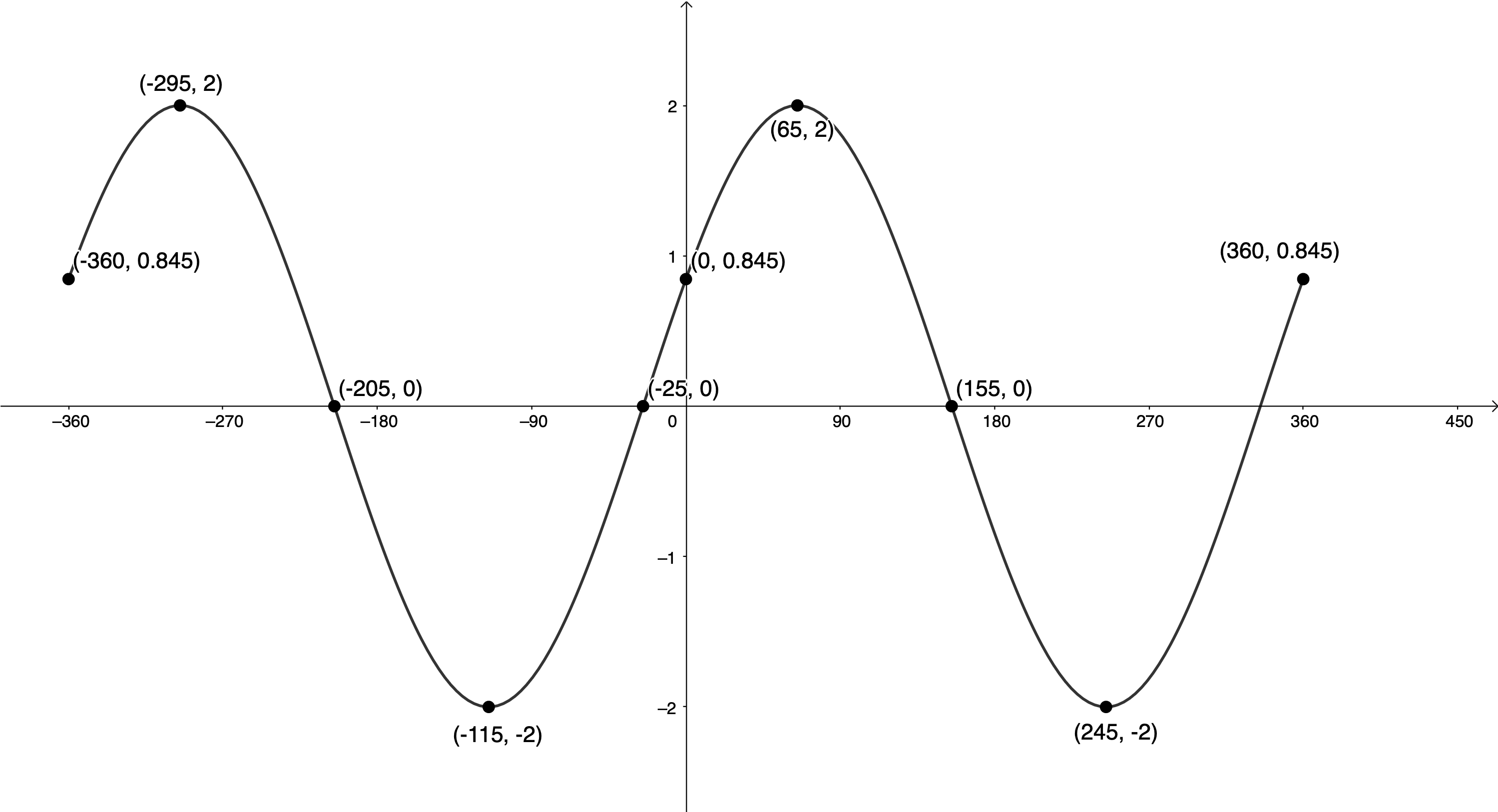
The function is of the form [latex]\scriptsize y=a\sin (x+p)[/latex] where [latex]\scriptsize a=2[/latex] and [latex]\scriptsize p={{25}^\circ}[/latex].
We need to transform one period of ‘anchor points’ noting that each of these points is going to be shifted [latex]\scriptsize {{25}^\circ}[/latex] to the left and each of the y-values is going to be multiplied by [latex]\scriptsize 2[/latex].
| [latex]\scriptsize \sin x[/latex] | [latex]\scriptsize ({{0}^\circ},0)[/latex] | [latex]\scriptsize ({{90}^\circ},1)[/latex] | [latex]\scriptsize ({{180}^\circ},0)[/latex] | [latex]\scriptsize ({{270}^\circ},-1)[/latex] | [latex]\scriptsize ({{360}^\circ},0)[/latex] |
| [latex]\scriptsize 2\sin (x+{{25}^\circ})[/latex] | [latex]\scriptsize (-{{25}^\circ},0)[/latex] | [latex]\scriptsize ({{65}^\circ},2)[/latex] | [latex]\scriptsize ({{155}^\circ},0)[/latex] | [latex]\scriptsize ({{245}^\circ},-2)[/latex] | [latex]\scriptsize ({{335}^\circ},0)[/latex] |
To make sure that the shape of the graph is as accurate as possible, we must also find the y-intercept.
y-intercept (let [latex]\scriptsize x=0[/latex]):
[latex]\scriptsize \begin{align*}y & =2\sin (0+{{25}^\circ})\\\therefore y & =0.845\end{align*}[/latex]
Because the period of the function is [latex]\scriptsize {{360}^\circ}[/latex] we know that the function values at [latex]\scriptsize -{{360}^\circ}[/latex] and [latex]\scriptsize {{360}^\circ}[/latex] will also be [latex]\scriptsize 0.845[/latex].
We can now plot our transformed ‘anchor points’ and draw the graph for the interval [latex]\scriptsize -{{360}^\circ}\le x\le {{360}^\circ}[/latex].
Example 8.3
Given the function [latex]\scriptsize f(x)=\sin ({{30}^\circ}-x)[/latex]:
- Sketch the graph for the interval [latex]\scriptsize {{0}^\circ}\le x\le {{360}^\circ}[/latex].
- State the intercepts with the axes.
- State the domain and range.
- State the amplitude and period.
Solutions
- The function is not in the form [latex]\scriptsize y=\sin (x+p)[/latex]. We first need to get it into this form.
[latex]\scriptsize \begin{align*}f(x)&=\sin ({{30}^\circ}-x)\\&=\sin (-x+{{30}^\circ})\\&=\sin \left( {-\left( {x-{{{30}}^\circ}} \right)} \right)\\&=-\sin (x-{{30}^\circ})\end{align*}[/latex]We know from the previous unit that [latex]\scriptsize \sin (-x)=-\sin (x)[/latex]. In other words, the graph of [latex]\scriptsize f(x)=\sin ({{30}^\circ}-x)[/latex] is going to be a reflection of the standard sine graph about the x-axis because [latex]\scriptsize a=-1[/latex].
.
We need to transform one period of ‘anchor points’ noting that each of these points is going to be shifted [latex]\scriptsize {{30}^\circ}[/latex] to the right and each of the y-values of these points needs to be multiplied by [latex]\scriptsize -1[/latex] .
.[latex]\scriptsize \sin x[/latex] [latex]\scriptsize ({{0}^\circ},0)[/latex] [latex]\scriptsize ({{90}^\circ},1)[/latex] [latex]\scriptsize ({{180}^\circ},0)[/latex] [latex]\scriptsize ({{270}^\circ},-1)[/latex] [latex]\scriptsize ({{360}^\circ},0)[/latex] [latex]\scriptsize \sin (x-{{30}^\circ})[/latex] [latex]\scriptsize ({{30}^\circ},0)[/latex] [latex]\scriptsize ({{120}^\circ},-1)[/latex] [latex]\scriptsize ({{210}^\circ},0)[/latex] [latex]\scriptsize ({{300}^\circ},1)[/latex] [latex]\scriptsize ({{390}^\circ},0)[/latex] .
To make sure that the shape of the graph is as accurate as possible, we must also find the y-intercept.
y-intercept (let [latex]\scriptsize x=0[/latex]):
[latex]\scriptsize \begin{align*}f(0) & =\sin ({{30}^\circ}-0)\\\therefore f(0) & =\displaystyle \frac{1}{2}\end{align*}[/latex]
.
Because the period of the function is [latex]\scriptsize {{360}^\circ}[/latex] we know that the function value at [latex]\scriptsize {{360}^\circ}[/latex] will also be [latex]\scriptsize \displaystyle \frac{1}{2}[/latex].
.
We can now plot our transformed ‘anchor points’ and draw the graph for the interval [latex]\scriptsize {{0}^\circ}\le x\le {{360}^\circ}[/latex].
.
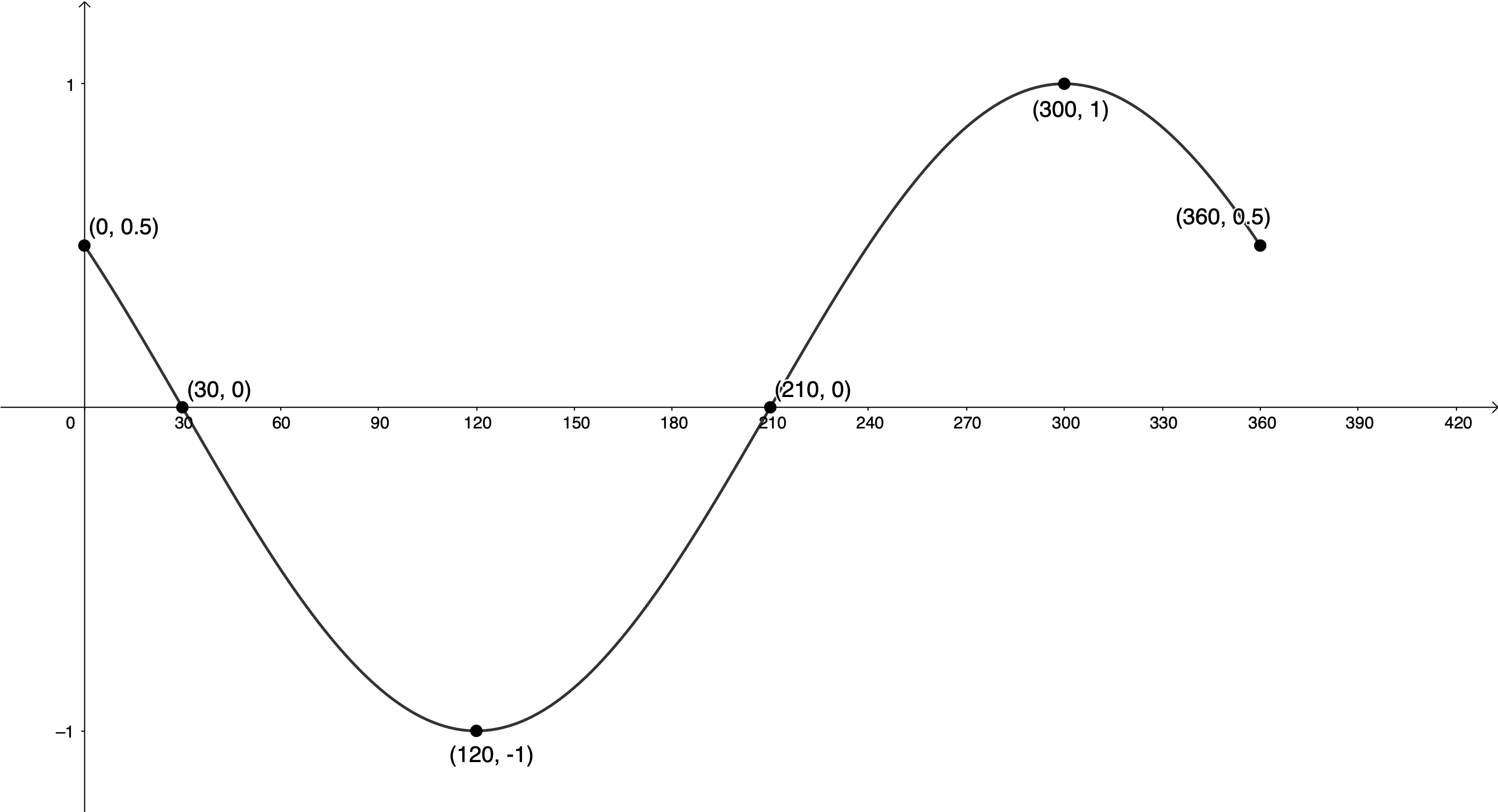
- y-intercept: [latex]\scriptsize ({{0}^\circ},\displaystyle \frac{1}{2})[/latex]
x-intercepts: [latex]\scriptsize ({{30}^\circ},0)[/latex] and [latex]\scriptsize ({{210}^\circ},0)[/latex] - Domain: [latex]\scriptsize \{x|x\in \mathbb{R},\text{ }{{0}^\circ}\le x\le {{360}^\circ}\}\text{ }[/latex]
Range: [latex]\scriptsize \{y|y\in \mathbb{R},\text{ }-1\le x\le 1\}\text{ }[/latex] - The period is [latex]\scriptsize {{360}^\circ}[/latex].
The amplitude is [latex]\scriptsize 1[/latex].
Exercise 8.2
For each of the following functions, sketch the graph for the indicated interval and state the domain and range:
- [latex]\scriptsize y=-2\sin (x+{{30}^\circ})[/latex] for [latex]\scriptsize -{{360}^\circ}\le x\le {{360}^\circ}[/latex]
- [latex]\scriptsize 2y=\sin (x+{{30}^\circ})[/latex] for [latex]\scriptsize {{0}^\circ}\le x\le {{360}^\circ}[/latex]
- [latex]\scriptsize \displaystyle \frac{1}{3}y=\sin ({{60}^\circ}-x)[/latex] for [latex]\scriptsize -{{360}^\circ}\le x\le {{360}^\circ}[/latex]
The full solutions are at the end of the unit.
Find the equation of a sine function of the form [latex]\scriptsize y=a\sin (x+p)[/latex]
If we examine the amplitude and the horizontal shift of [latex]\scriptsize y=a\sin (x+p)[/latex], we can determine the values of [latex]\scriptsize a[/latex] and [latex]\scriptsize p[/latex].
Example 8.4
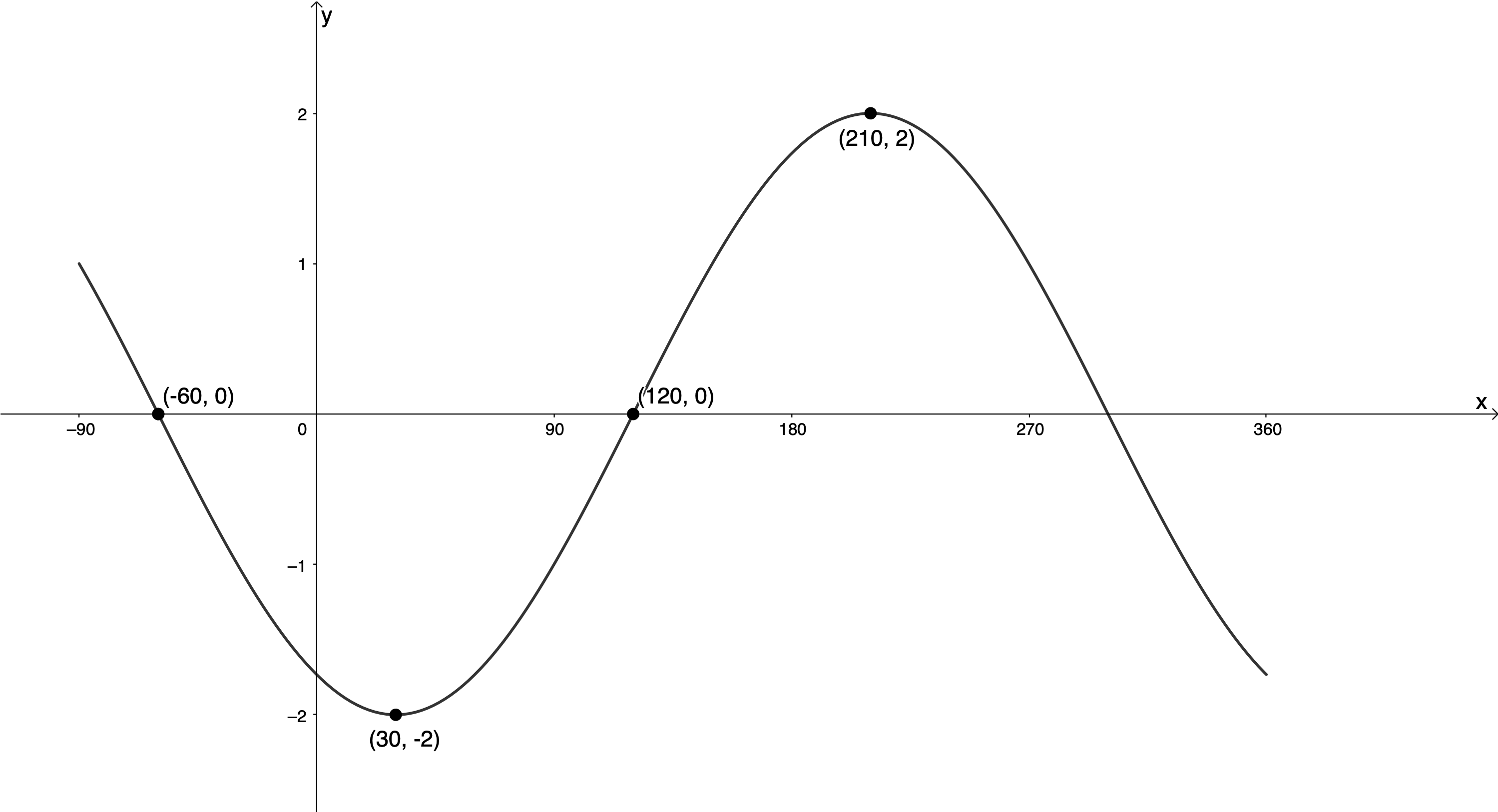
The graph below is a function of the form [latex]\scriptsize y=a\sin (x+p)[/latex]. Determine the values of [latex]\scriptsize a[/latex] and [latex]\scriptsize p[/latex].
Solution
We are told that the function is of the form [latex]\scriptsize y=a\sin (x+p)[/latex].
Firstly, we can see that the amplitude of the graph is [latex]\scriptsize 2[/latex] units. We do not yet know whether [latex]\scriptsize a=2[/latex] or [latex]\scriptsize a=-2[/latex].
We know that the function [latex]\scriptsize y=\sin x[/latex] passes through the origin and then reaches a maximum turning point at [latex]\scriptsize ({{90}^\circ},1)[/latex]. This function instead reaches a minimum turning point at [latex]\scriptsize ({{30}^\circ},-2)[/latex]. Therefore, we can say that the point [latex]\scriptsize ({{90}^\circ},1)[/latex] has been transformed into the point [latex]\scriptsize ({{30}^\circ},-2)[/latex].
This means that the point has been shifted horizontally [latex]\scriptsize {{60}^\circ}[/latex] to the left and the y-value has been multiplied by [latex]\scriptsize -2[/latex]. Therefore, [latex]\scriptsize a=-2[/latex] and [latex]\scriptsize p={{60}^\circ}[/latex], and the function is [latex]\scriptsize y=-2\sin (x+{{60}^\circ})[/latex].
Exercise 8.3
Given the graph of the form [latex]\scriptsize y=a\sin (x+p)[/latex]:
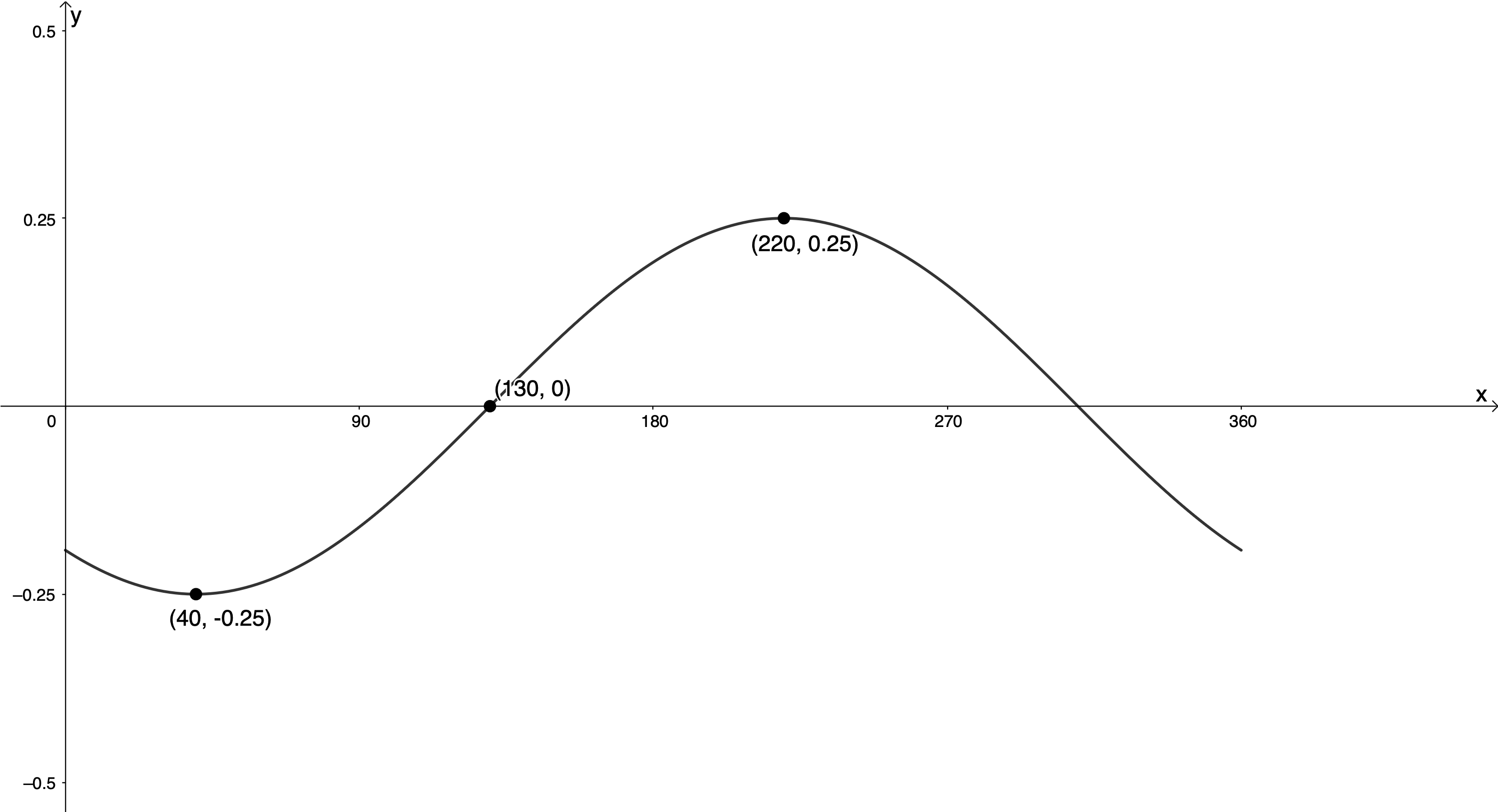
- Determine the values of [latex]\scriptsize a[/latex] and [latex]\scriptsize p[/latex].
- State the domain and range of the function.
The full solutions are at the end of the unit.
Summary
In this unit you have learnt the following:
- The effects of [latex]\scriptsize a[/latex] and [latex]\scriptsize p[/latex] on the sine graph of the form [latex]\scriptsize y=a\sin (x+p)[/latex].
- How to sketch functions of the form[latex]\scriptsize y=a\sin (x+p)[/latex].
- How to find the values of [latex]\scriptsize a[/latex] and [latex]\scriptsize p[/latex] from a given sine graph of the form[latex]\scriptsize y=a\sin (x+p)[/latex].
Unit 8: Assessment
Suggested time to complete: 45 minutes
- Sketch the following functions for the given intervals:
- [latex]\scriptsize 3y=\sin \left( {x-{{{45}}^\circ}} \right)[/latex] for [latex]\scriptsize -{{360}^\circ}\le x\le {{360}^\circ}[/latex]
- [latex]\scriptsize g(x)=-4\sin ({{60}^\circ}-x)[/latex] for [latex]\scriptsize {{0}^\circ}\le x\le {{360}^\circ}[/latex]
- From the graph below of the form [latex]\scriptsize y=a\sin (x+p)[/latex], determine the values of [latex]\scriptsize a[/latex] and [latex]\scriptsize p[/latex].
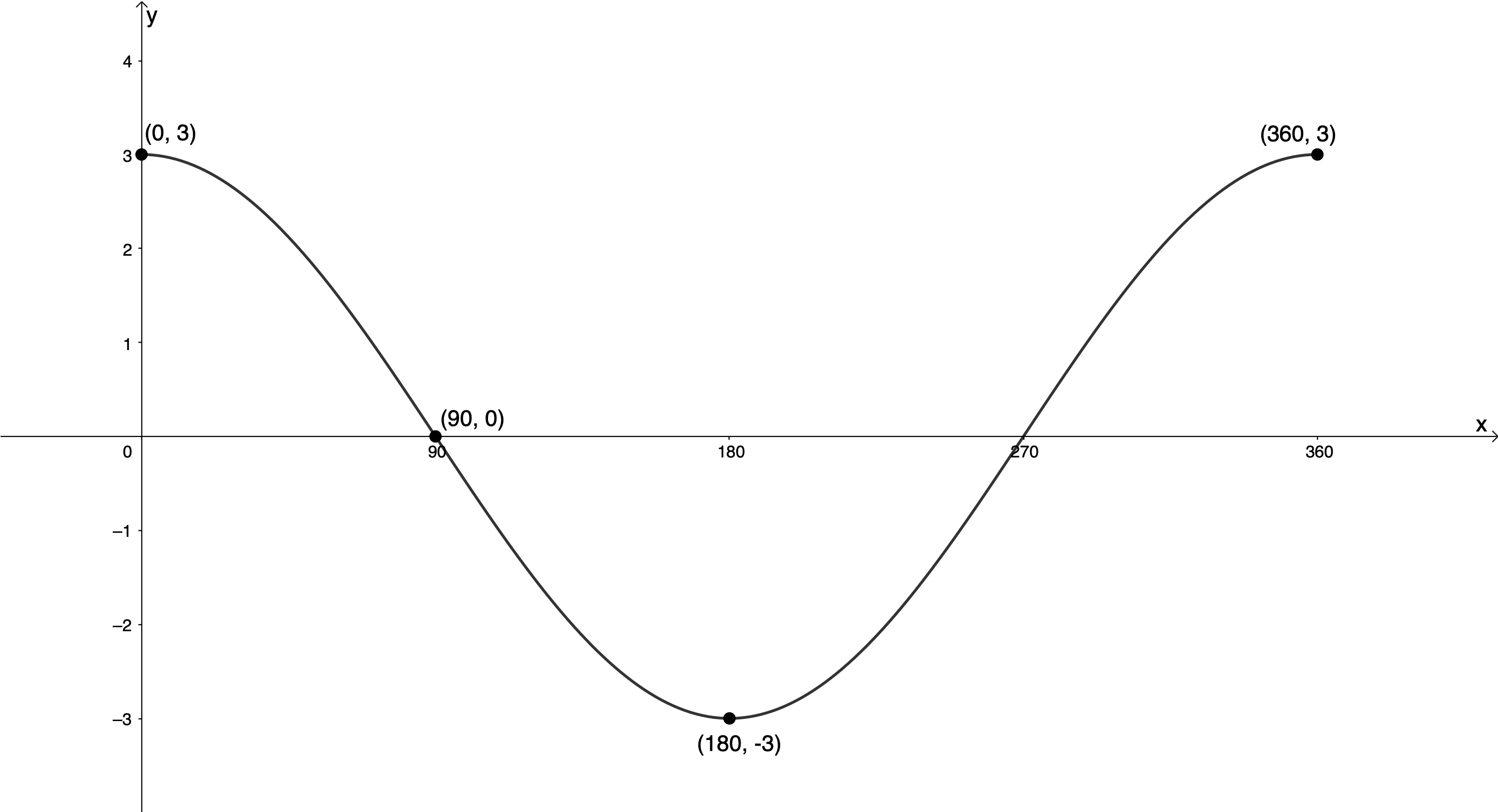
The full solutions are at the end of the unit.
Unit 8: Solutions
Exercise 8.1
- [latex]\scriptsize f(x)=\sin (x+{{30}^\circ})[/latex] for [latex]\scriptsize {{0}^\circ}\le x\le {{360}^\circ}[/latex]
[latex]\scriptsize p={{30}^\circ}[/latex]. Therefore, the graph will be shifted [latex]\scriptsize {{30}^\circ}[/latex] to the left.[latex]\scriptsize \sin x[/latex] [latex]\scriptsize ({{0}^\circ},0)[/latex] [latex]\scriptsize ({{90}^\circ},1)[/latex] [latex]\scriptsize ({{180}^\circ},0)[/latex] [latex]\scriptsize ({{270}^\circ},-1)[/latex] [latex]\scriptsize ({{360}^\circ},0)[/latex] [latex]\scriptsize \sin (x+{{30}^\circ})[/latex] [latex]\scriptsize (-{{30}^\circ},0)[/latex] [latex]\scriptsize ({{60}^\circ},1)[/latex] [latex]\scriptsize ({{150}^\circ},0)[/latex] [latex]\scriptsize ({{240}^\circ},-1)[/latex] [latex]\scriptsize ({{330}^\circ},0)[/latex] .
y-intercept (let [latex]\scriptsize x=0[/latex]):
[latex]\scriptsize \begin{align*}f(0) & =\sin ({{0}^\circ}+{{30}^\circ})\\\therefore f(0) & =\displaystyle \frac{1}{2}\end{align*}[/latex]
.
Because the period of the function is [latex]\scriptsize {{360}^\circ}[/latex] we know that the function value at [latex]\scriptsize {{360}^\circ}[/latex] will also be [latex]\scriptsize \displaystyle \frac{1}{2}[/latex].
.

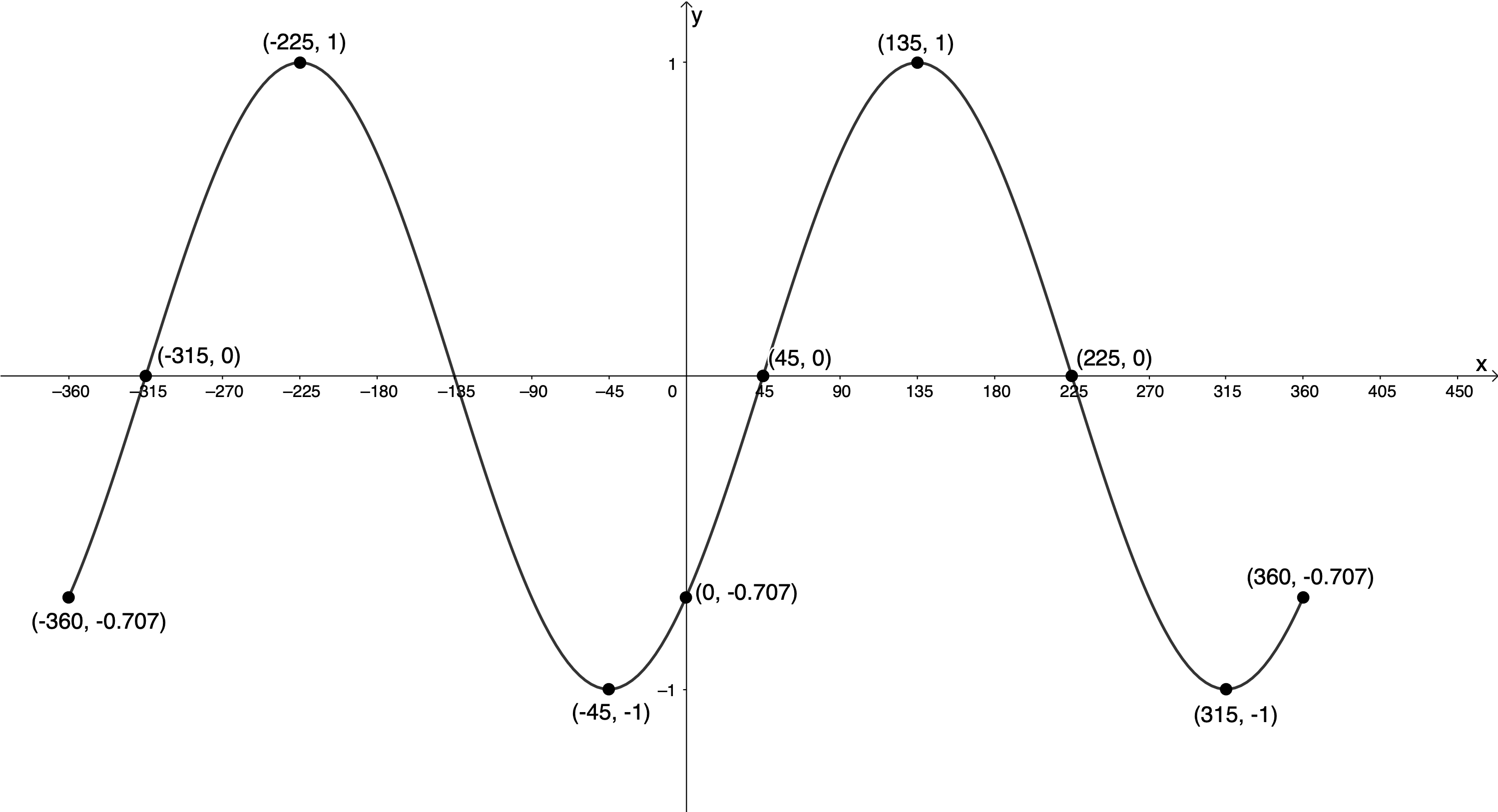
- [latex]\scriptsize g(x)=\sin (x-{{45}^\circ})[/latex] for [latex]\scriptsize -{{360}^\circ}\le x\le {{360}^\circ}[/latex]
[latex]\scriptsize p=-{{45}^\circ}[/latex]. Therefore, the graph will be shifted [latex]\scriptsize {{45}^\circ}[/latex] to the right.[latex]\scriptsize \sin x[/latex] [latex]\scriptsize ({{0}^\circ},0)[/latex] [latex]\scriptsize ({{90}^\circ},1)[/latex] [latex]\scriptsize ({{180}^\circ},0)[/latex] [latex]\scriptsize ({{270}^\circ},-1)[/latex] [latex]\scriptsize ({{360}^\circ},0)[/latex] [latex]\scriptsize \sin (x-{{45}^\circ})[/latex] [latex]\scriptsize ({{45}^\circ},0)[/latex] [latex]\scriptsize ({{135}^\circ},1)[/latex] [latex]\scriptsize ({{225}^\circ},0)[/latex] [latex]\scriptsize ({{315}^\circ},-1)[/latex] [latex]\scriptsize ({{405}^\circ},0)[/latex] .
y-intercept (let [latex]\scriptsize x=0[/latex]):
[latex]\scriptsize \begin{align*}g(0) & =\sin ({{0}^\circ}-{{45}^\circ})\\\therefore g(0) & =-\displaystyle \frac{1}{{\sqrt{2}}}=-0.707\end{align*}[/latex]
.
Because the period of the function is [latex]\scriptsize {{360}^\circ}[/latex] we know that the function values at [latex]\scriptsize -{{360}^\circ}[/latex] and [latex]\scriptsize {{360}^\circ}[/latex] will also be [latex]\scriptsize -0.707[/latex].
.
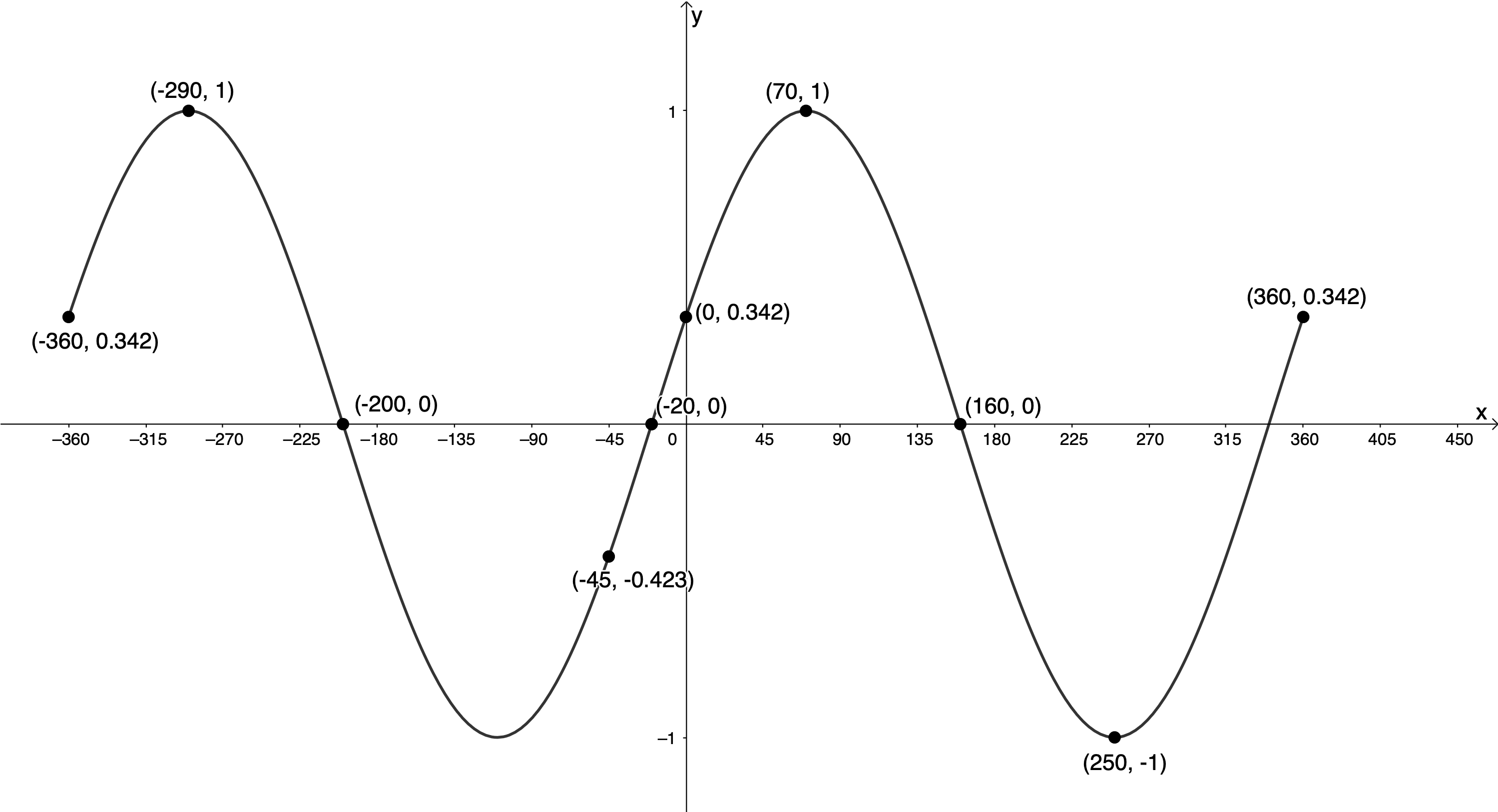
- [latex]\scriptsize h(x)=\sin (x+{{20}^\circ})[/latex] for [latex]\scriptsize -{{360}^\circ}\le x\le {{360}^\circ}[/latex]
[latex]\scriptsize p={{20}^\circ}[/latex]. Therefore, the graph will be shifted [latex]\scriptsize {{20}^\circ}[/latex] to the left.[latex]\scriptsize \sin x[/latex] [latex]\scriptsize ({{0}^\circ},0)[/latex] [latex]\scriptsize ({{90}^\circ},1)[/latex] [latex]\scriptsize ({{180}^\circ},0)[/latex] [latex]\scriptsize ({{270}^\circ},-1)[/latex] [latex]\scriptsize ({{360}^\circ},0)[/latex] [latex]\scriptsize \sin (x+{{20}^\circ})[/latex] [latex]\scriptsize (-{{20}^\circ},0)[/latex] [latex]\scriptsize ({{70}^\circ},1)[/latex] [latex]\scriptsize ({{160}^\circ},0)[/latex] [latex]\scriptsize ({{250}^\circ},-1)[/latex] [latex]\scriptsize ({{340}^\circ},0)[/latex] .
y-intercept (let [latex]\scriptsize x=0[/latex]):
[latex]\scriptsize \begin{align*}h(0) & =\sin ({{0}^\circ}+{{20}^\circ})\\\therefore h(0) & =0.342\end{align*}[/latex]
.
Because the period of the function is [latex]\scriptsize {{360}^\circ}[/latex] we know that the function values at [latex]\scriptsize -{{360}^\circ}[/latex] and [latex]\scriptsize {{360}^\circ}[/latex] will also be [latex]\scriptsize 0.342[/latex].
.
Exercise 8.2
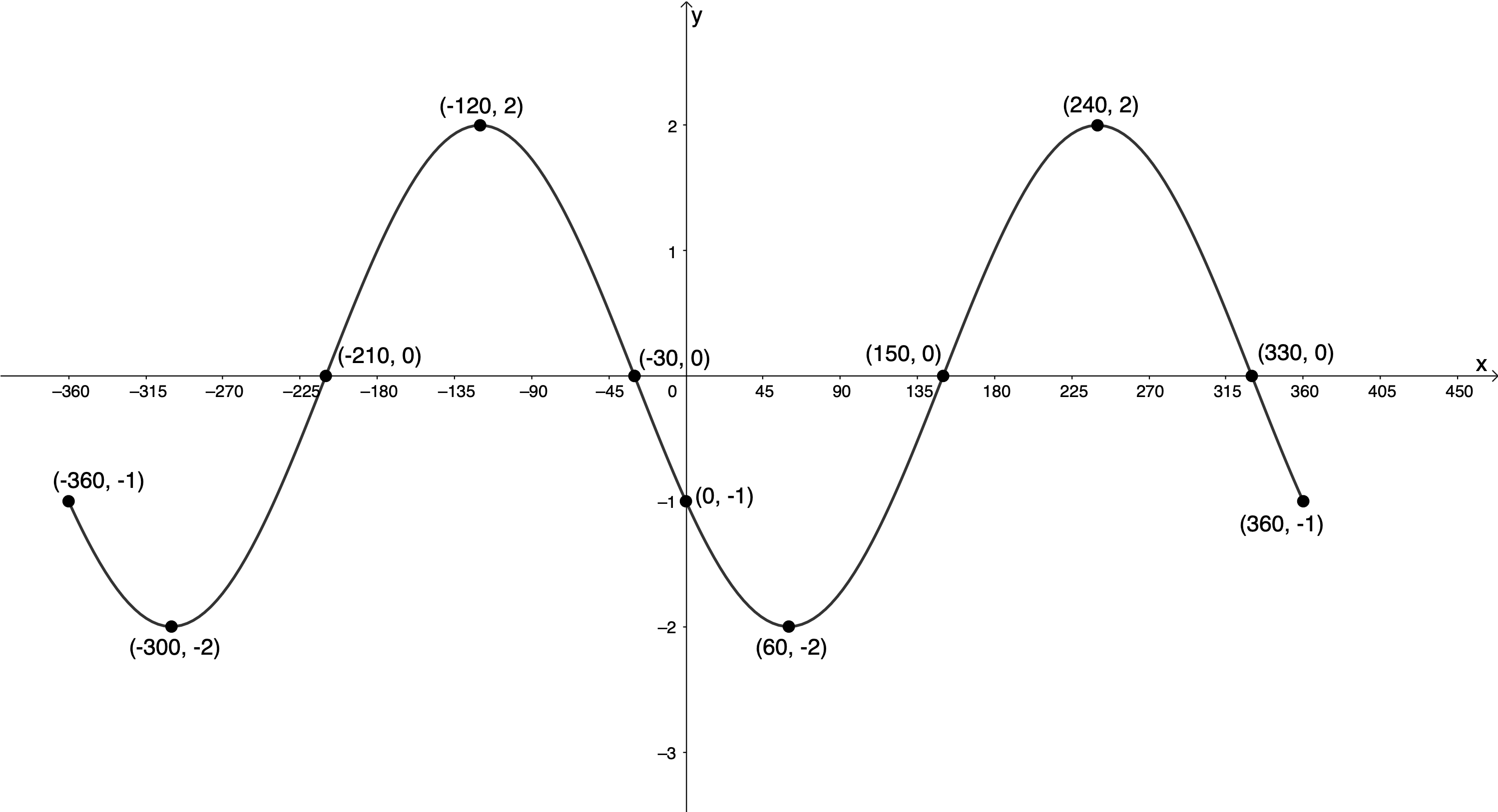
- [latex]\scriptsize y=-2\sin (x+{{30}^\circ})[/latex] for [latex]\scriptsize -{{360}^\circ}\le x\le {{360}^\circ}[/latex]
[latex]\scriptsize a=-2[/latex]. Therefore, the amplitude will be [latex]\scriptsize 2[/latex] and the graph will be reflected about the x-axis.
[latex]\scriptsize p={{30}^\circ}[/latex]. Therefore, the graph will be shifted [latex]\scriptsize {{30}^\circ}[/latex] to the left.[latex]\scriptsize \sin x[/latex] [latex]\scriptsize ({{0}^\circ},0)[/latex] [latex]\scriptsize ({{90}^\circ},1)[/latex] [latex]\scriptsize ({{180}^\circ},0)[/latex] [latex]\scriptsize ({{270}^\circ},-1)[/latex] [latex]\scriptsize ({{360}^\circ},0)[/latex] [latex]\scriptsize -2\sin (x+{{30}^\circ})[/latex] [latex]\scriptsize (-{{30}^\circ},0)[/latex] [latex]\scriptsize ({{60}^\circ},-2)[/latex] [latex]\scriptsize ({{150}^\circ},0)[/latex] [latex]\scriptsize ({{240}^\circ},2)[/latex] [latex]\scriptsize ({{330}^\circ},0)[/latex] .
y-intercept (let [latex]\scriptsize x=0[/latex]):
[latex]\scriptsize \begin{align*}y & =-2\sin ({{0}^\circ}+{{30}^\circ})\\\therefore y & =-1\end{align*}[/latex]
.
Because the period of the function is [latex]\scriptsize {{360}^\circ}[/latex] we know that the function values at [latex]\scriptsize -{{360}^\circ}[/latex] and [latex]\scriptsize {{360}^\circ}[/latex] will also be [latex]\scriptsize -1[/latex].
.
.
Domain: [latex]\scriptsize \{x|x\in \mathbb{R},\text{ }-{{360}^\circ}\le x\le {{360}^\circ}\}[/latex]
Range: [latex]\scriptsize \{y|y\in \mathbb{R},\text{ }-2\le y\le 2\}[/latex] - [latex]\scriptsize 2y=\sin (x+{{30}^\circ})[/latex] for [latex]\scriptsize {{0}^\circ}\le x\le {{360}^\circ}[/latex]
[latex]\scriptsize \begin{align*}2y & =\sin (x+{{30}^\circ})\\\therefore y & =\displaystyle \frac{1}{2}\sin (x+{{30}^\circ})\end{align*}[/latex]
[latex]\scriptsize a=\displaystyle \frac{1}{2}[/latex]. Therefore, the amplitude will be [latex]\scriptsize \displaystyle \frac{1}{2}[/latex].
[latex]\scriptsize p={{30}^\circ}[/latex]. Therefore, the graph will be shifted [latex]\scriptsize {{30}^\circ}[/latex] to the left.[latex]\scriptsize \sin x[/latex] [latex]\scriptsize ({{0}^\circ},0)[/latex] [latex]\scriptsize ({{90}^\circ},1)[/latex] [latex]\scriptsize ({{180}^\circ},0)[/latex] [latex]\scriptsize ({{270}^\circ},-1)[/latex] [latex]\scriptsize ({{360}^\circ},0)[/latex] [latex]\scriptsize \displaystyle \frac{1}{2}\sin (x+{{30}^\circ})[/latex] [latex]\scriptsize (-{{30}^\circ},0)[/latex] [latex]\scriptsize ({{60}^\circ},\displaystyle \frac{1}{2})[/latex] [latex]\scriptsize ({{150}^\circ},0)[/latex] [latex]\scriptsize ({{240}^\circ},-\displaystyle \frac{1}{2})[/latex] [latex]\scriptsize ({{330}^\circ},0)[/latex] .
y-intercept (let [latex]\scriptsize x=0[/latex]):
[latex]\scriptsize \begin{align*}y & =\displaystyle \frac{1}{2}\sin ({{0}^\circ}+{{30}^\circ})\\\therefore y & =\displaystyle \frac{1}{4}\end{align*}[/latex]
.
Because the period of the function is [latex]\scriptsize {{360}^\circ}[/latex] we know that the function value at [latex]\scriptsize {{360}^\circ}[/latex] will also be [latex]\scriptsize \displaystyle \frac{1}{4}[/latex].
.
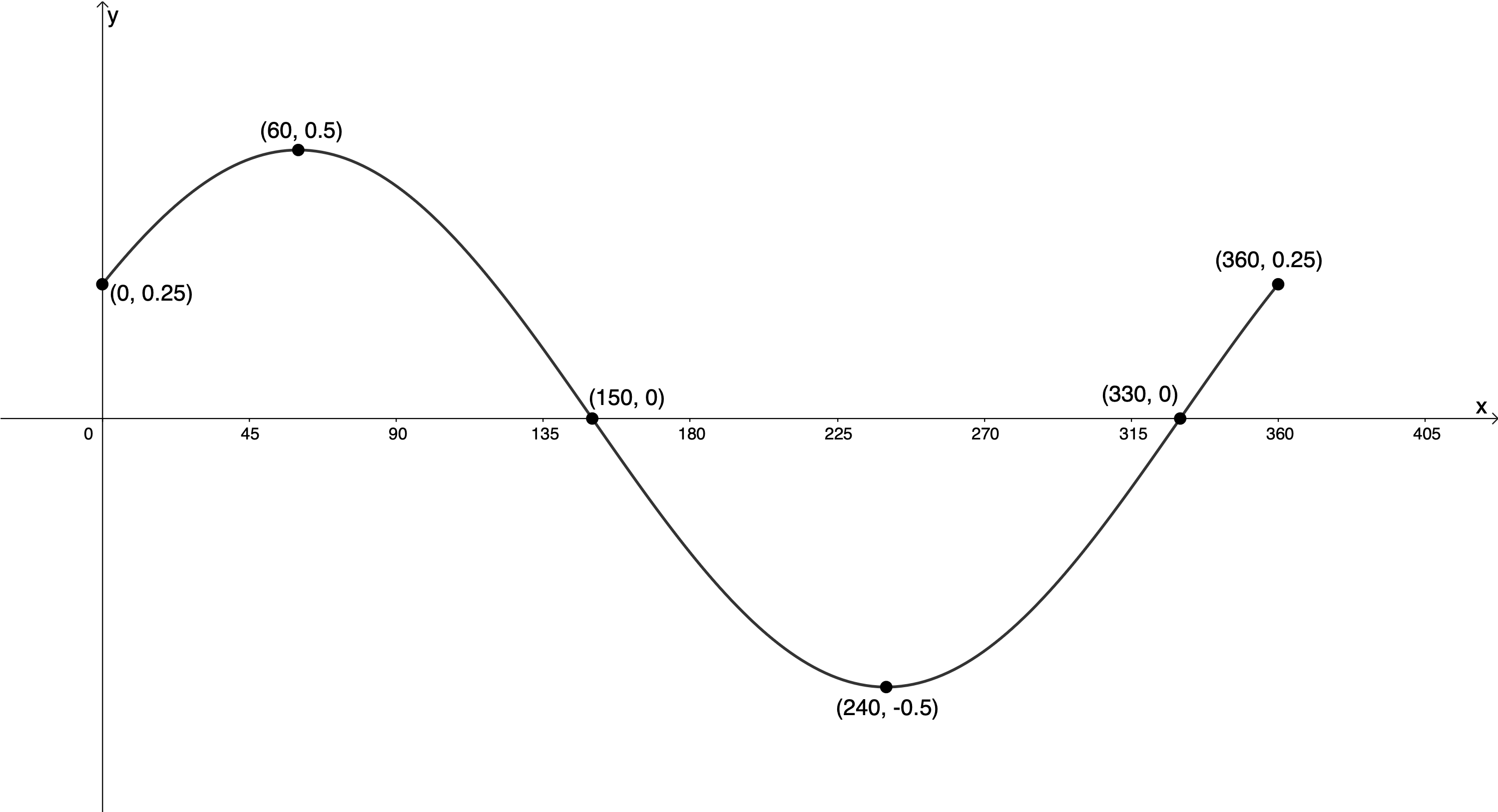
.
Domain: [latex]\scriptsize \{x|x\in \mathbb{R},\text{ }{{0}^\circ}\le x\le {{360}^\circ}\}[/latex]
Range: [latex]\scriptsize \{y|y\in \mathbb{R},\text{ }-\displaystyle \frac{1}{2}\le y\le \displaystyle \frac{1}{2}\}[/latex] - [latex]\scriptsize \displaystyle \frac{1}{3}y=\sin ({{60}^\circ}-x)[/latex] for [latex]\scriptsize -{{360}^\circ}\le x\le {{360}^\circ}[/latex]
[latex]\scriptsize \begin{align*}\displaystyle \frac{1}{3}y & =\sin ({{60}^\circ}-x)\\\therefore y & =3\sin ({{60}^\circ}-x)\\&=3\sin (-x+{{60}^\circ})\\&=3\sin \left( {-\left( {x-{{{60}}^\circ}} \right)} \right)\\&=-3\sin (x-{{60}^\circ})\end{align*}[/latex]
[latex]\scriptsize a=-3[/latex]. Therefore, the amplitude will be [latex]\scriptsize 3[/latex] and the graph will be reflected about the x-axis.
[latex]\scriptsize p=-{{60}^\circ}[/latex]. Therefore, the graph will be shifted [latex]\scriptsize {{60}^\circ}[/latex] to the right.[latex]\scriptsize \sin x[/latex] [latex]\scriptsize ({{0}^\circ},0)[/latex] [latex]\scriptsize ({{90}^\circ},1)[/latex] [latex]\scriptsize ({{180}^\circ},0)[/latex] [latex]\scriptsize ({{270}^\circ},-1)[/latex] [latex]\scriptsize ({{360}^\circ},0)[/latex] [latex]\scriptsize -3\sin (x-{{60}^\circ})[/latex] [latex]\scriptsize ({{60}^\circ},0)[/latex] [latex]\scriptsize ({{150}^\circ},-3)[/latex] [latex]\scriptsize ({{240}^\circ},0)[/latex] [latex]\scriptsize ({{330}^\circ},3)[/latex] [latex]\scriptsize ({{420}^\circ},0)[/latex] .
y-intercept (let [latex]\scriptsize x=0[/latex]):
[latex]\scriptsize \begin{align*}y & =-3\sin ({{0}^\circ}-{{60}^\circ})\\\therefore y & =-3\times -\displaystyle \frac{{\sqrt{3}}}{2}=2,598\end{align*}[/latex]
.
Because the period of the function is [latex]\scriptsize {{360}^\circ}[/latex] we know that the function values at [latex]\scriptsize -{{360}^\circ}[/latex] and [latex]\scriptsize {{360}^\circ}[/latex] will also be [latex]\scriptsize 2.598[/latex].
.
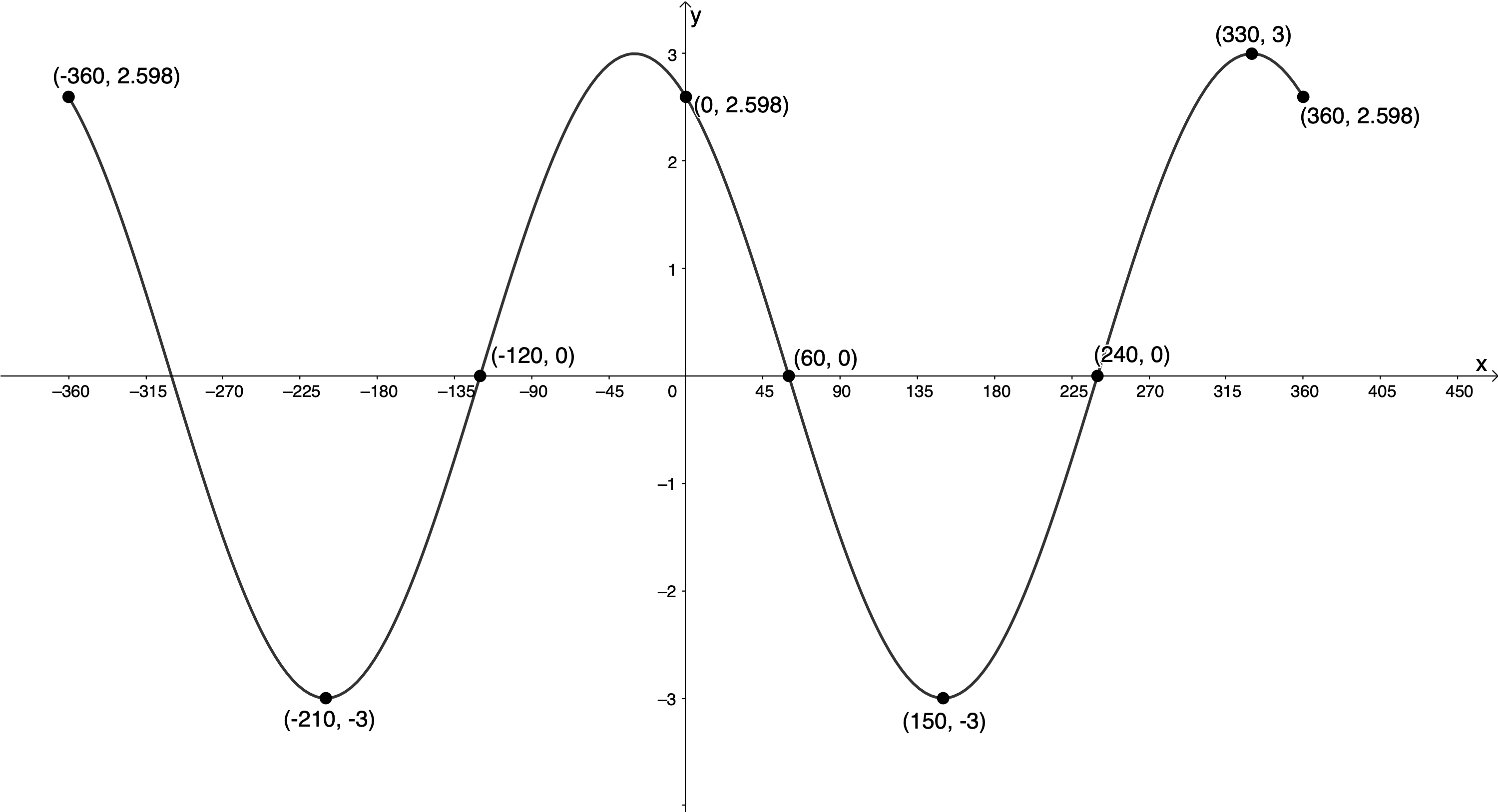
.
Domain: [latex]\scriptsize \{x|x\in \mathbb{R},\text{ }-{{360}^\circ}\le x\le {{360}^\circ}\}[/latex]
Range: [latex]\scriptsize \{y|y\in \mathbb{R},\text{ }-3\le y\le 3\}[/latex]
Exercise 8.3
- Amplitude is [latex]\scriptsize 0.25=\displaystyle \frac{1}{4}[/latex]
[latex]\scriptsize ({{90}^\circ},1)[/latex] has been transformed to [latex]\scriptsize ({{40}^\circ},-\displaystyle \frac{1}{4})[/latex]. Therefore, [latex]\scriptsize a=-\displaystyle \frac{1}{4}[/latex] and [latex]\scriptsize p={{50}^\circ}[/latex].
[latex]\scriptsize y=-\displaystyle \frac{1}{4}\sin (x+{{50}^\circ})[/latex] - Domain: [latex]\scriptsize \{x|x\in \mathbb{R},\text{ }{{0}^\circ}\le x\le {{360}^\circ}\}[/latex]
Range: [latex]\scriptsize \{y|y\in \mathbb{R},\text{ }-\displaystyle \frac{1}{4}\le y\le \displaystyle \frac{1}{4}\}[/latex]
Unit 8: Assessment
- .
- [latex]\scriptsize 3y=\sin \left( {x-{{{45}}^\circ}} \right)[/latex] for [latex]\scriptsize -{{360}^\circ}\le x\le {{360}^\circ}[/latex]
[latex]\scriptsize \begin{align*}3y & =\sin (x-{{45}^\circ})\\\therefore y & =\displaystyle \frac{1}{3}\sin (x-{{45}^\circ})\end{align*}[/latex]
[latex]\scriptsize a=\displaystyle \frac{1}{3}[/latex]. Therefore, the amplitude will be [latex]\scriptsize \displaystyle \frac{1}{3}[/latex].
[latex]\scriptsize p=-{{45}^\circ}[/latex]. Therefore, the graph will be shifted [latex]\scriptsize {{45}^\circ}[/latex] to the right.[latex]\scriptsize \sin x[/latex] [latex]\scriptsize ({{0}^\circ},0)[/latex] [latex]\scriptsize ({{90}^\circ},1)[/latex] [latex]\scriptsize ({{180}^\circ},0)[/latex] [latex]\scriptsize ({{270}^\circ},-1)[/latex] [latex]\scriptsize ({{360}^\circ},0)[/latex] [latex]\scriptsize \displaystyle \frac{1}{3}\sin (x-{{45}^\circ})[/latex] [latex]\scriptsize ({{45}^\circ},0)[/latex] [latex]\scriptsize ({{135}^\circ},\displaystyle \frac{1}{3})[/latex] [latex]\scriptsize ({{225}^\circ},0)[/latex] [latex]\scriptsize ({{315}^\circ},-\displaystyle \frac{1}{3})[/latex] [latex]\scriptsize ({{405}^\circ},0)[/latex] .
y-intercept (let [latex]\scriptsize x=0[/latex]):
[latex]\scriptsize \begin{align*}y & =\displaystyle \frac{1}{3}\sin ({{0}^\circ}-{{45}^\circ})\\\therefore y & =\displaystyle \frac{1}{3}\times -\displaystyle \frac{1}{{\sqrt{2}}}=-0.236\end{align*}[/latex]
.
Because the period of the function is [latex]\scriptsize {{360}^\circ}[/latex] we know that the function values at [latex]\scriptsize -{{360}^\circ}[/latex] and [latex]\scriptsize {{360}^\circ}[/latex] will also be [latex]\scriptsize 0.236[/latex].
.
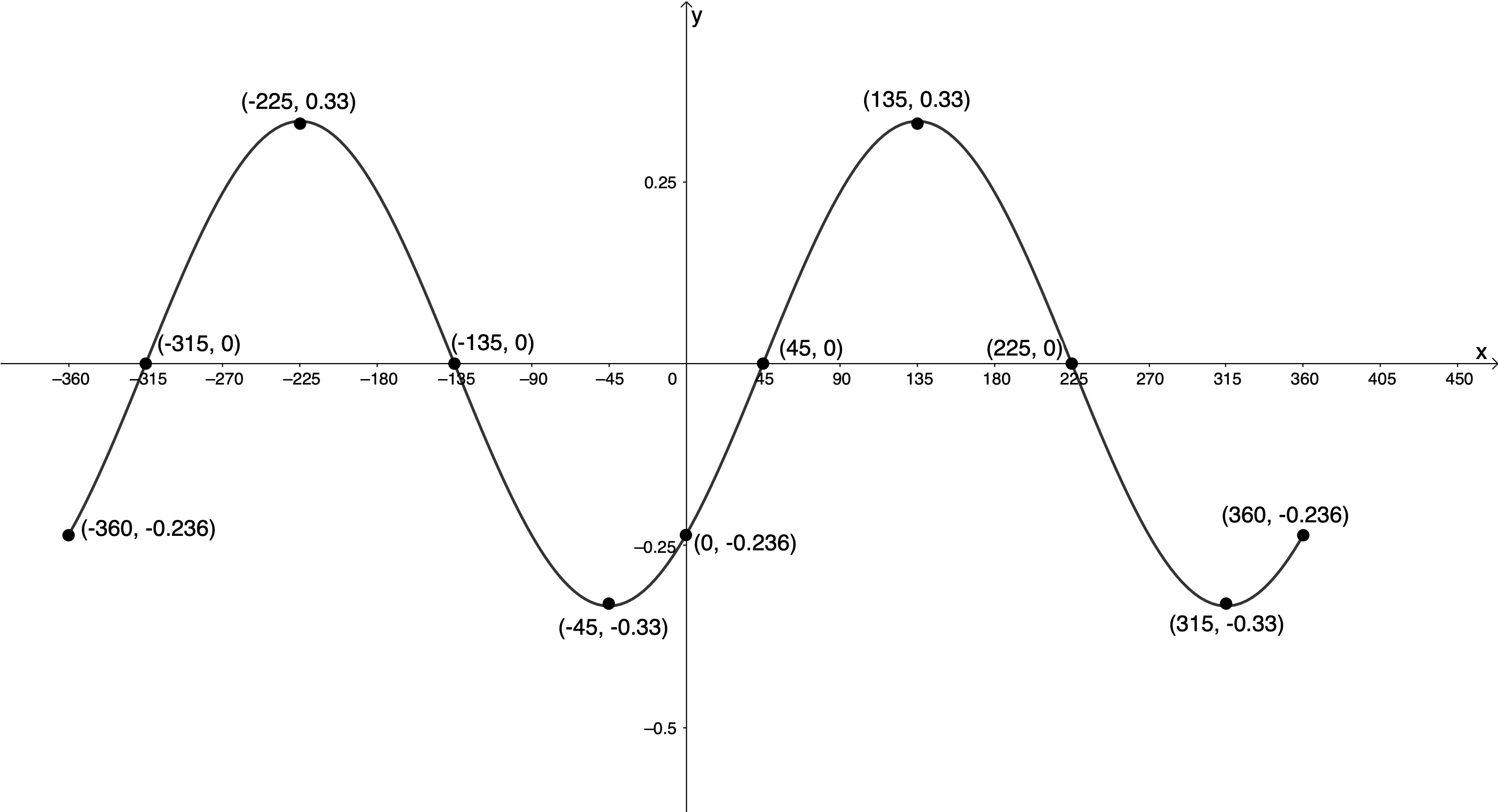
- [latex]\scriptsize g(x)=-4\sin ({{60}^\circ}-x)[/latex] for [latex]\scriptsize {{0}^\circ}\le x\le {{360}^\circ}[/latex]
[latex]\scriptsize \begin{align*}g(x) & =-4\sin ({{60}^\circ}-x)\\&=-4\sin (-x+{{60}^\circ})\\&=-4\sin \left( {-\left( {x-{{{60}}^\circ}} \right)} \right)\\&=4\sin (x-{{60}^\circ})\end{align*}[/latex]
[latex]\scriptsize a=4[/latex]. Therefore, the amplitude will be [latex]\scriptsize 4[/latex].
[latex]\scriptsize p=-{{60}^\circ}[/latex]. Therefore, the graph will be shifted [latex]\scriptsize {{60}^\circ}[/latex] to the right.[latex]\scriptsize \sin x[/latex] [latex]\scriptsize ({{0}^\circ},0)[/latex] [latex]\scriptsize ({{90}^\circ},1)[/latex] [latex]\scriptsize ({{180}^\circ},0)[/latex] [latex]\scriptsize ({{270}^\circ},-1)[/latex] [latex]\scriptsize ({{360}^\circ},0)[/latex] [latex]\scriptsize 4\sin (x+{{60}^\circ})[/latex] [latex]\scriptsize ({{60}^\circ},0)[/latex] [latex]\scriptsize ({{150}^\circ},4)[/latex] [latex]\scriptsize ({{240}^\circ},0)[/latex] [latex]\scriptsize ({{330}^\circ},-4)[/latex] [latex]\scriptsize ({{420}^\circ},0)[/latex] .
y-intercept (let [latex]\scriptsize x=0[/latex]):
[latex]\scriptsize \begin{align*}g(0)&=4\sin (0-{{60}^\circ})\\\therefore y & =4\times -\displaystyle \frac{{\sqrt{3}}}{2}=-3.464\end{align*}[/latex]
.
Because the period of the function is [latex]\scriptsize {{360}^\circ}[/latex] we know that the function value at [latex]\scriptsize {{360}^\circ}[/latex] will also be [latex]\scriptsize -3.464[/latex].
.
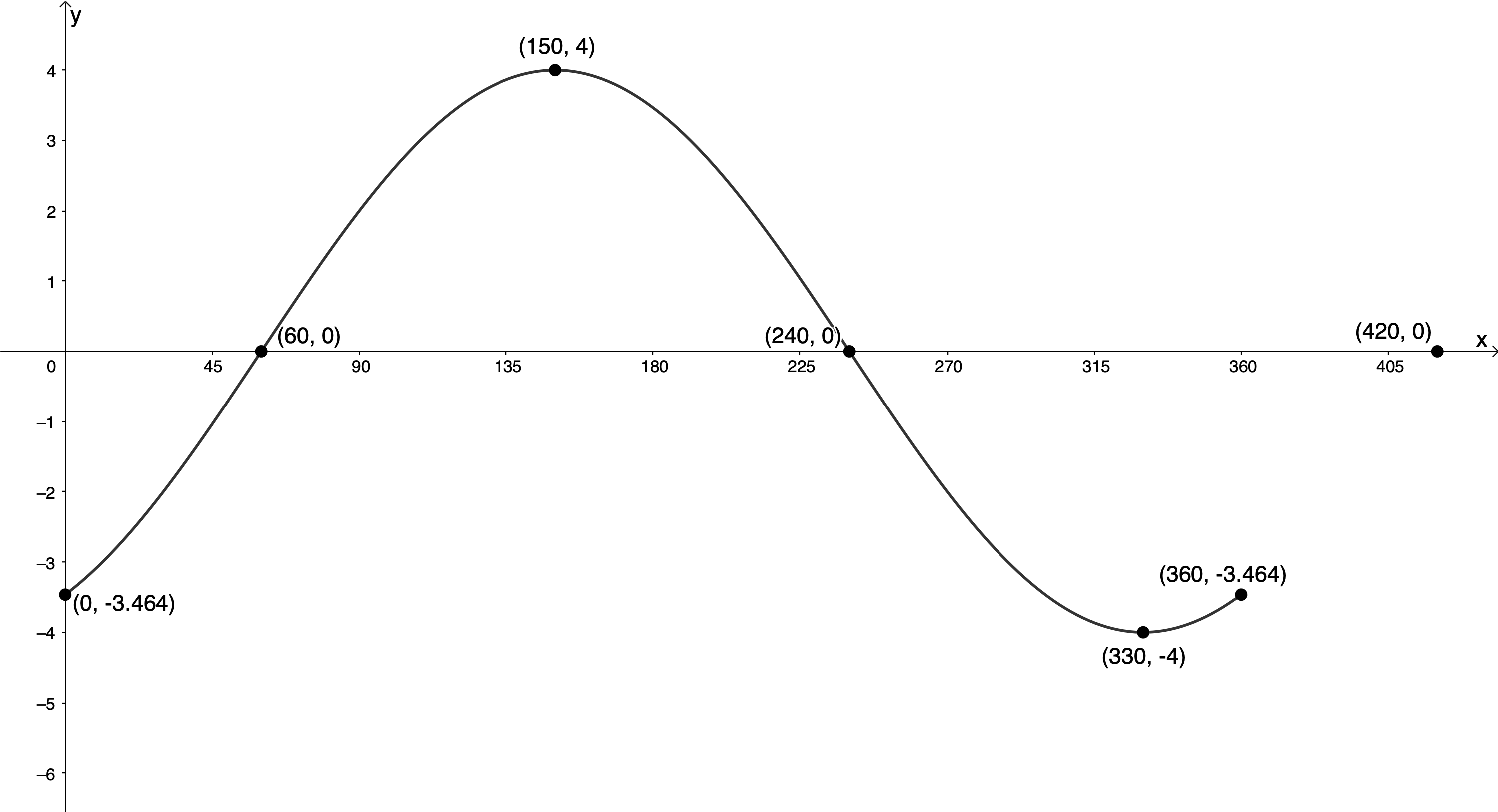
- [latex]\scriptsize 3y=\sin \left( {x-{{{45}}^\circ}} \right)[/latex] for [latex]\scriptsize -{{360}^\circ}\le x\le {{360}^\circ}[/latex]
- The function is of the form [latex]\scriptsize y=a\sin (x+p)[/latex]. The amplitude is [latex]\scriptsize 3[/latex]. The point [latex]\scriptsize ({{90}^\circ},1)[/latex] has been transformed to [latex]\scriptsize ({{0}^\circ},3)[/latex]. Therefore, the graph has been shifted [latex]\scriptsize {{90}^\circ}[/latex]to the left. Hence [latex]\scriptsize a=3[/latex] and [latex]\scriptsize p={{90}^\circ}[/latex] and the function is [latex]\scriptsize y=3\sin (x+{{90}^\circ})[/latex].
Media Attributions
- figure1 © Geogebra is licensed under a CC BY-SA (Attribution ShareAlike) license
- figure2 © Geogebra is licensed under a CC BY-SA (Attribution ShareAlike) license
- figure3 © Geogebra is licensed under a CC BY-SA (Attribution ShareAlike) license
- figure4 © Geogebra is licensed under a CC BY-SA (Attribution ShareAlike) license
- takenote © DHET is licensed under a CC BY (Attribution) license
- example8.1 © Geogebra is licensed under a CC BY-SA (Attribution ShareAlike) license
- example8.2 © Geogebra is licensed under a CC BY-SA (Attribution ShareAlike) license
- example8.3 © Geogebra is licensed under a CC BY-SA (Attribution ShareAlike) license
- example8.4 © Geogebra is licensed under a CC BY-SA (Attribution ShareAlike) license
- exercise8.3 © Geogebra is licensed under a CC BY-SA (Attribution ShareAlike) license
- assessmentQ2 © Geogebra is licensed under a CC BY-SA (Attribution ShareAlike) license
- exercise8.1A1 © Geogebra is licensed under a CC BY-SA (Attribution ShareAlike) license
- exercise8.1A2 © Geogebra is licensed under a CC BY-SA (Attribution ShareAlike) license
- exercise8.1A3 © Geogebra is licensed under a CC BY-SA (Attribution ShareAlike) license
- exercise8.2A1 © Geogebra is licensed under a CC BY-SA (Attribution ShareAlike) license
- exercise8.2A2 © Geogebra is licensed under a CC BY-SA (Attribution ShareAlike) license
- exercise8.2A3 © Geogebra is licensed under a CC BY-SA (Attribution ShareAlike) license
- assessmentA1a © Geogebra is licensed under a CC BY-SA (Attribution ShareAlike) license
- assessmentA1b © Geogebra is licensed under a CC BY-SA (Attribution ShareAlike) license
